|
Peremennye Zvezdy (Variable Stars) 42, No. 1, 2022 Received 1 February; accepted 16 February. |
Article in PDF |
|
DOI: 10.24412/2221-0474-2022-42-1-7
|
V961 Cep: A New Eclipsing Variable with a 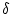 Sct
Component
Sct
Component
Igor Volkov1,2
- Sternberg Astronomical Institute, Lomonosov Moscow
University, Universitetsky Ave., 13, 119992 Moscow, Russia
- Institute of Astronomy of the Russian Academy of Sciences, Pyatnitskaya str., 48, 119017 Moscow, Russia
|
New observations of V961 Cep, an eclipsing star with
elliptical orbit (
|
1. Introduction
The star V961 Cep was first studied in detail in Volkov et al.
(2010). The main characteristics of the component stars were
determined, and it was found that the primary component was
located at the boundary of the instability strip for ![]() Sct
variables in the Hertzsprung-Russell(HR) diagram. However, no
intrinsic variability was detected at that time.
Sct
variables in the Hertzsprung-Russell(HR) diagram. However, no
intrinsic variability was detected at that time.
2. Observations and analysis
Our observations were continued with the Zeiss-1000 and Zeiss-600
telescopes of Simeiz INASAN observatory using ![]() photometers with CCD VersArray 512UV and FLI PL09000 detectors.
photometers with CCD VersArray 512UV and FLI PL09000 detectors.
The main goal of our observational program is to study the
internal structure of stars by measuring the rate of apsidal
motion in elliptical systems (Volkov and Volkova, 2009). Thus, the
observations were mainly carried out in minima. There was concern
because of the shape of several minima being distorted and the
accuracy of timing being much worse than in other our studies
(Bagaev et al., 2018). For a long time, we could not figure out
the reason. There can be several explanations for poor accuracy:
intrinsic variability of one of the comparison stars; intrinsic
variability of V961 Cep itself; instrumental reasons related to
the fact that nearby comparison stars are too bright for
observations with 0.6-1-meter apertures and CCDs, see Table 1 in
Volkov et al. (2010). The problem was resolved when we had
monitored the star in the secondary minimum and in quadrature at
the INASAN Zvenigorod observatory in autumn, 2021 with a
relatively small automatic telescope, Celestron RASA ![]() ,
equipped with the ZWO ASI 6200 MM PRO camera. We used comparison
stars not so bright as in our previous study: GSC 4479 446 was the
primary standard and GSC 4479 771, the check star. The results of
this monitoring in the quadrature in
,
equipped with the ZWO ASI 6200 MM PRO camera. We used comparison
stars not so bright as in our previous study: GSC 4479 446 was the
primary standard and GSC 4479 771, the check star. The results of
this monitoring in the quadrature in ![]() band are presented in
Figs. 1, 2.
band are presented in
Figs. 1, 2.
 |
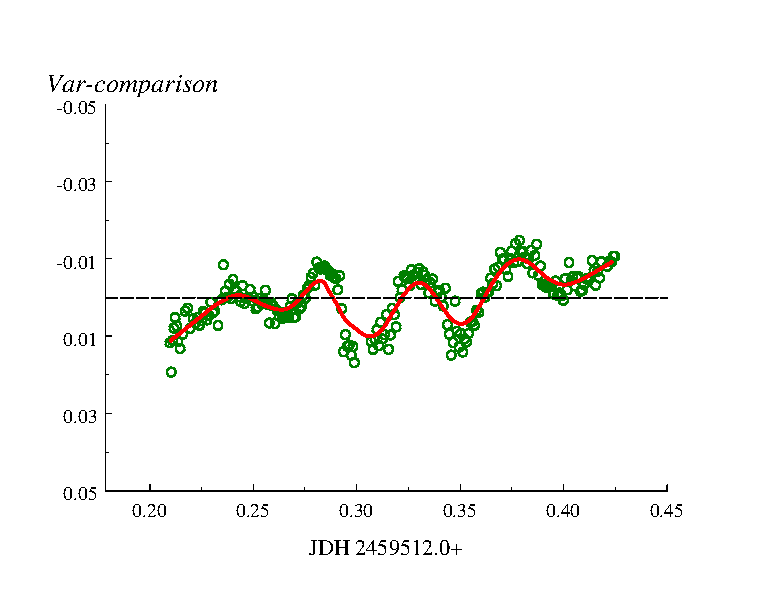
Fig. 1. Monitoring of V961 Cep in quadrature. To control the stability of the atmosphere and equipment, the same graph for the check star is shown below. |
The accuracy of measurements was
![]() on both nights. We
clearly see a
on both nights. We
clearly see a ![]() Sct-like oscillation with a period a little
longer than 1 hour. At first we were not sure that the accuracy of
our previous measurements was sufficient to search for the period
of the oscillations over the entire observational set. In
addition, we found that there was a third body in the system
orbiting the eclipsing star with a period of 18 years - the LIght
Time Effect (LITE) is clearly seen in our new minima timings. We
discussed such a possibility in our first paper because the
addition of a third light slightly improved the accuracy of the
Light Curve (LC) solution. However, at that time, we did not have
at our disposal a sufficiently long observational series as we
have now. The study of the LITE has not yet been completed and
will not be discussed here. We would just like to say that, having
corrected our observations for the LITE, we made an attempt to
clarify the value of the pulsation period and to check its
stability. Since most of our observations were made in minima, we
removed eclipses from the LC with the help of our program that
implements the algorithm described in Khaliullin and Khaliullina
(1984). Observations with increased scatter were excluded from the
analysis. Finally, we obtained an array of homogeneous
Sct-like oscillation with a period a little
longer than 1 hour. At first we were not sure that the accuracy of
our previous measurements was sufficient to search for the period
of the oscillations over the entire observational set. In
addition, we found that there was a third body in the system
orbiting the eclipsing star with a period of 18 years - the LIght
Time Effect (LITE) is clearly seen in our new minima timings. We
discussed such a possibility in our first paper because the
addition of a third light slightly improved the accuracy of the
Light Curve (LC) solution. However, at that time, we did not have
at our disposal a sufficiently long observational series as we
have now. The study of the LITE has not yet been completed and
will not be discussed here. We would just like to say that, having
corrected our observations for the LITE, we made an attempt to
clarify the value of the pulsation period and to check its
stability. Since most of our observations were made in minima, we
removed eclipses from the LC with the help of our program that
implements the algorithm described in Khaliullin and Khaliullina
(1984). Observations with increased scatter were excluded from the
analysis. Finally, we obtained an array of homogeneous ![]() observations containing 684 points covering 13 years. We find that
pulsations do not fully disappear in the primary minimum: partial
eclipses occur in this minimum. The amplitude of pulsations
changes a little, as it is clearly seen in Figs. 1, 2.
observations containing 684 points covering 13 years. We find that
pulsations do not fully disappear in the primary minimum: partial
eclipses occur in this minimum. The amplitude of pulsations
changes a little, as it is clearly seen in Figs. 1, 2.
3. Implementation of our program of period determination
We use our program of period determination that implements an algorithm for enumerating trial period values in a given interval with a fixed step using the sliding average. For each value of the period in given interval, the phases of each observation point are found. The initial epoch is set arbitrarily. The array is then sorted by phases using the quick sort algorithm (Knuth, 1973). After such a preparation of the array, we calculate deviations from the mean for each point. To calculate the mean value for each point, we take several points before and after the point of interest. Then we find the expected value of the point of interest by linear interpolation between the two mean points, before and after the point of interest. The difference between calculated and observed values is interpreted as the observational error. After finishing this procedure, the mean error for all points in the observational array is calculated and recorded in an archive file along with the trial period value. Then, the trial period is increased by a fixed step, and the procedure is repeated. After finishing the enumerating process, the resulting table is presented as a graph: error - trial period. The extreme values on this plot are interpreted as possible periods of brightness variation. Normally, we have a set of day and year aliases. In the case of V961 Per, one can expect aliases with the orbital period of the star because we mainly observed in minima.
If a period exists, the method simultaneously gives the mean error
of the observations. When the period is unknown, we use a wide
interval of trial periods searching with relatively rough steps.
After identification of the area of interest, we use a more
detailed grid. The result for V961 Cep is presented in Fig. 3
(preliminary search) and Fig. 4 (detailed investigation). The mean
error of observations is
![]() . There are many artificial
7-day aliases to the orbital period of variable.
. There are many artificial
7-day aliases to the orbital period of variable.
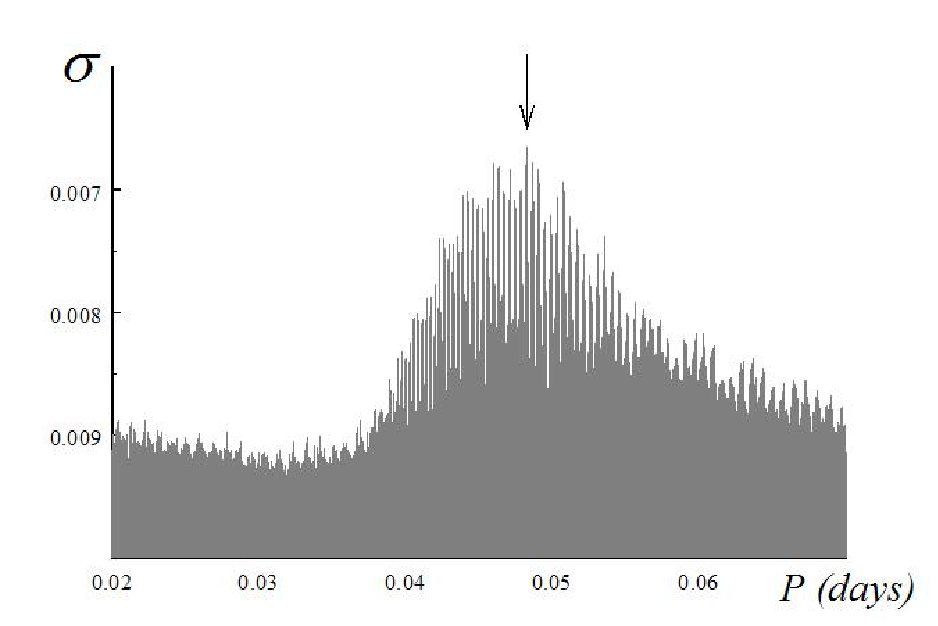 |
Fig. 3. The periodogram of the preliminary period search. The arrow indicated the accepted hump of possible periods. |
We carefully reviewed the convolutions of observations with periods corresponding to the maxima in Figs. 3, 4 and chose the following ephemeris for the times of maximum brightness:
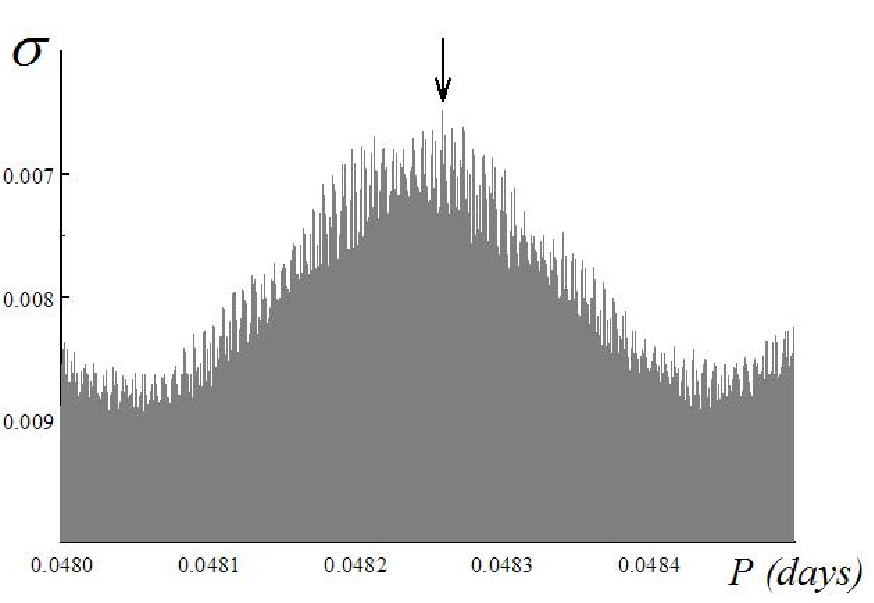 |
Fig. 4. The detailed periodogram in the accepted hump area. The arrow indicates the best period value derived by inspection of the best promising periods with respect to contradictions for individual nights. |
This ephemeris has no contradiction with individual nights of
observations. The LC phased with this period is shown in Fig. 5.
In future calculations of brightness maxima, one should keep in
mind that the amplitude of the LITE is up to 10 minutes, so the
times of maximum can be shifted within these limits. The full
pulsation amplitude is only
![]()
![]() , see Fig. 5. The real
amplitude is almost twice larger, because the brightness of the
primary component is close to 60% of the brightness of the triple
system.
, see Fig. 5. The real
amplitude is almost twice larger, because the brightness of the
primary component is close to 60% of the brightness of the triple
system.
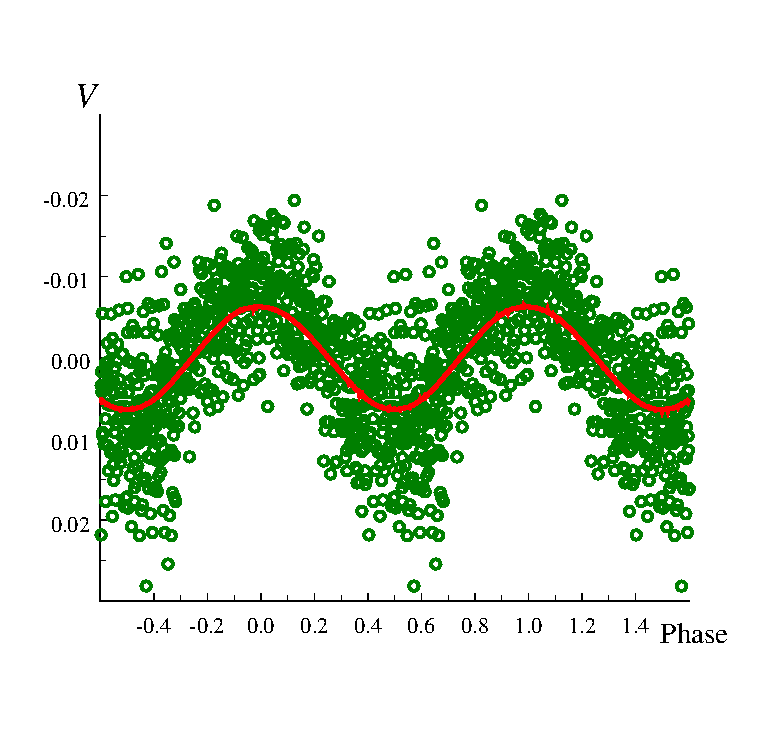 |
Fig. 5.
Observations of the star accumulated
during 13 years, phased with
|
The pulsation period found by us has been stable for 13 years. The
star is similar to V577 Oph (F0 V+F1 V) whose primary component is
a ![]() Scuti pulsator and the system is also triple (Volkov,
1990; Volkov and Volkova, 2010; Volkov, 2015; Jeffery et al.,
2017). The HR diagram for eclipsing stars with pulsators presented
in Fig. 6 was taken from Soydugan et al.(2006). The positions of
the stars V961 Cep and V577 Oph are shown as red circles. The
primary components of both stars are at the red border of the
instability strip. However, the age and sizes of V577 Oph are
larger than those for V961 Cep, and its period (
Scuti pulsator and the system is also triple (Volkov,
1990; Volkov and Volkova, 2010; Volkov, 2015; Jeffery et al.,
2017). The HR diagram for eclipsing stars with pulsators presented
in Fig. 6 was taken from Soydugan et al.(2006). The positions of
the stars V961 Cep and V577 Oph are shown as red circles. The
primary components of both stars are at the red border of the
instability strip. However, the age and sizes of V577 Oph are
larger than those for V961 Cep, and its period (
![]() )
is longer. The temperatures of the stars were derived according to
the calibration from Flower (1996), which gives higher
temperatures than the Popper (1980) calibration used in our
previous work. Higher temperatures put V577 Oph definitely inside
the instability strip, with V961 Cep exactly at its red boundary,
so Flower's calibrations are better suited for accurate analysis.
)
is longer. The temperatures of the stars were derived according to
the calibration from Flower (1996), which gives higher
temperatures than the Popper (1980) calibration used in our
previous work. Higher temperatures put V577 Oph definitely inside
the instability strip, with V961 Cep exactly at its red boundary,
so Flower's calibrations are better suited for accurate analysis.
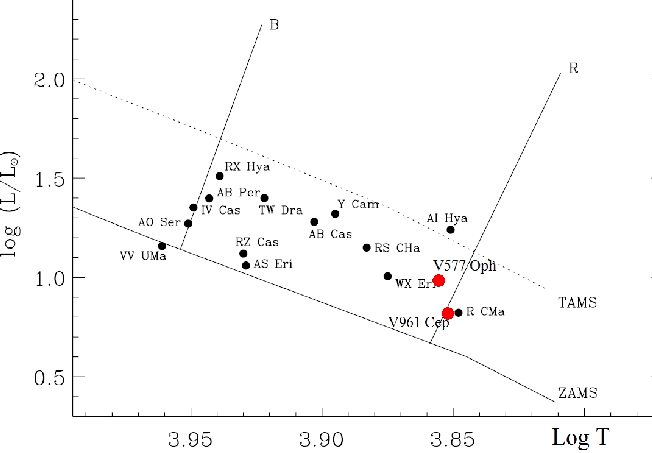 |
Fig. 6.
Positions of the |
Refinement of the temperature scale also reveals that the position
of V961 Cep in the HR diagram almost completely coincides with the
position of the main component of R CMa (Sp=F0 V). It was very
interesting to find that the periods and amplitudes of pulsations
of both stars were very close; for R CMa,
![]() and amplitude
and amplitude
![]() (Mkrtichian
and Gamarova, 2000). This may indicate that the basic physical
characteristics of the two stars are also identical. Besides
exhibiting pulsations, R CMa is also a triple system
(Radhakrishnan et al., 1984).
(Mkrtichian
and Gamarova, 2000). This may indicate that the basic physical
characteristics of the two stars are also identical. Besides
exhibiting pulsations, R CMa is also a triple system
(Radhakrishnan et al., 1984).
4. Conclusions
We have established that V961 Cep belongs to a small class of very interesting eclipsing stars with a pulsating component. We plan to continue observations in order to correct the model of the star for the presence of the third body, not taken into account in our first study.
Acknowledgements. This study was supported by the SAIA (Slovakia) scholarship.
References:
Bagaev, L. A., Volkov, I. M., and Nikolenko, I. V., 2018, Astron. Rep., 62, 664
Flower, P. J., 1996, Astrophys. J., 469, 355
Jeffery, E. J., Barnes, T. G. III, Skillen, I., and Montemayor, T. J., 2017, Astron. J., 154, 127
Khaliullina, A. I. and Khaliullin, Kh. F., 1984, Sov. Astron., 28, 228
Knuth, D., 1998, In: The Art of Computer Programming, 3, Reading, Massachusetts: Addison-Wesley
Mkrtichian, D. E. and Gamarova, A. Yu., 2000, Inform. Bull. Var. Stars, No.4836
Popper, D. M., 1980, Ann. Rev. Astron. Astrophys., 18, 115
Radhakrishnan, K. R., Sarma, M. B. K. and Abhyankar, K. D., 1984, Astrophys. Space Sci., 99, 229
Soydugan, E., Soydugan, F., Demircan, O. and Ibanoglu, C., 2006, Mon. Not. Roy.Astron. Soc., 370, 2013
Volkov, I. M., 1990, Inform. Bull. Var. Stars, No.3493
Volkov, I. M., 2015, in ASP Conf. Series, 496, Living Together: Planets, Host Stars and Binaries, ed. S. M. Rucinski, G. Torres, and M. Zejda (San Francisco, CA: ASP), 109
Volkov, I. M. and Volkova, N. S., 2009, Astron. Rep., 53, 136
Volkov, I. M. and Volkova, N. S., 2010, ASP Conf. Ser., 435, 323
Volkov, I. M., Volkova, N. S., and Chochol, D., 2010, Astron. Rep., 54, 418