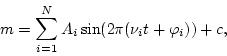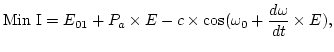|
Peremennye Zvezdy (Variable Stars) 42, No. 4, 2022 Received 20 May; accepted 8 June. |
Article in PDF |
|
DOI: 10.24412/2221-0474-2022-42-17-27
|
Photometric Elements, Apsidal Motion, Brightness Variations, and Light-time Effect in the Eclipsing Binary System V957 Cephei
V. S. Kozyreva1, A. V. Kusakin2, A. I. Bogomazov1, Ch. T. Omarov2, A. V. Krylov1
- P.K. Sternberg Astronomical Institute, M.V. Lomonosov
Moscow State University, 119234, Universitetskij prospect, 13,
Moscow, Russia
- V.G. Fesenkov Astrophysical Institute, National Space
Agency, 050020, Observatory, 23, Almaty,
Kazakhstan
We obtained several light curves of V957 Cep in 2009,
2011, 2013, 2016, and 2019 at the Crimean Astronomical Station of
M.V. Lomonosov Moscow State University and at the Tien Shan
Observatory of the V.G. Fesenkov Astrophysical Institute. Using
the TESS satellite observations, we found brightness variations
of V957 Cep with the period
 and amplitude
 . For our light curves (2009-2019) and
TESS light curves (2019-2020), orbital elements were computed and
a new value of the apsidal motion rate
 yr was derived. We found a possible
light-time effect that can indicate gravitational influence of one
or several additional bodies in the system on the central binary.
Based on the currently available data, the amplitude and period of
the light-time effect still cannot be reliably estimated.
|
1. Introduction
V957 Cep was recognized as an eclipsing variable (its orbital
period being 1
98873) in an analysis of NSVS survey data on
variable stars in the northern hemisphere (Wozniak et al.,
2004), and it was included in the list of 50 new eclipsing stars
with elliptical orbits found in data of ASAS, Hipparcos, and NSVS
(Otero et al., 2006).
We obtained the variable's light curves in the 
band at the
Tien Shan Astronomical Observatory of the V.G. Fesenkov
Astrophysical Institute (in 2009, 2011 using the 35-cm
Ritchey-Chrétien telescope with an ST-402 CCD; in 2013, 2016,
2019 using the 1-m Zeiss telescope with an Apogee 49000D9 CCD; in
2016, 2019 using the 60-cm Zeiss telescope with an Apogee Aspen
CCD). Studies of the light curves, parameters of the components,
orbital elements, and the determination of the apsidal motion rate
were published by Kozyreva et al. (2012), Kozyreva & Kusakin
(2014).
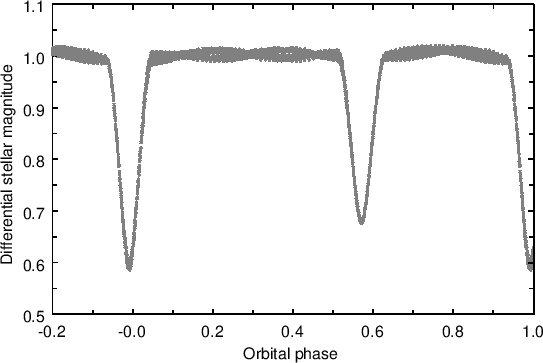
The system was also observed in two series of observations by the TESS space telescope in 2019-2020, the data were taken from the MAST archive1. The precision of TESS observations is about a few thousandths of a magnitude, it is several times better than the precision of ground-based observations. Figure 1 shows the combined light curve of the system obtained by TESS in 2019-2020.
The spectral types of the components were estimated as B7V-A3V using spectrophotometric observations made in Barnesville near Washington using an 18-inch Newton telescope (Kozyreva et al., 2012).
2. Analysis of TESS light curve pulsations
We analysed TESS observations using the PERDET code, based on the Fourier analysis (Breger, 1990), outside minima and obtained a frequency analysis of pulsations of the object's luminosity in the following form:
where 
is the differential magnitude of the object;
, the number of pulsations; 
, time; 
is the amplitude
of pulsations; 
, the frequency of pulsations; 
is the initial phase of pulsations, and 
is the normalizing
constant.
, d
, d
, mag
0.015
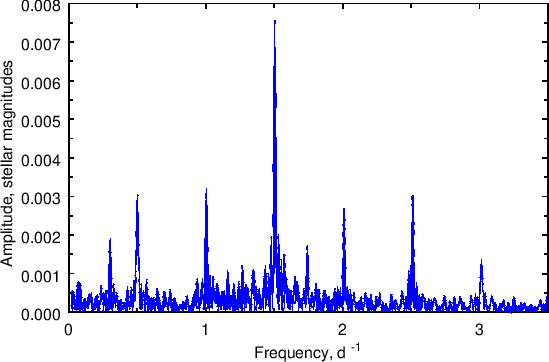
Figure 2 shows the spectral amplitude, its highest peak
corresponds to the frequency
d and
amplitude
.
The reliability of the pulsations can be characterized by
, where 
is the
root-mean-square error of initial observations, 
is the
root-mean-square error obtained after subtraction of the pulsation
(Stellingwerf, 1978). The lower is this criterion, the higher is
the reliability of the process. For the
d
pulsation, the criterion is
, so
the reliability is reasonably high. Table 1 shows parameters of
the pulsation.
 |
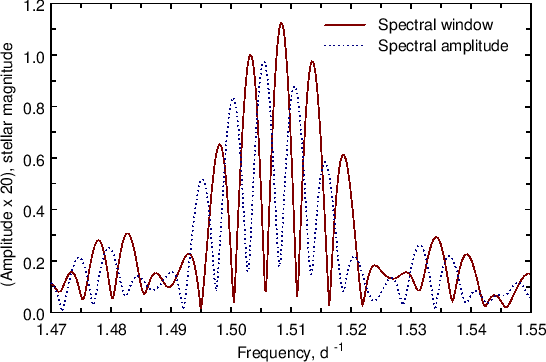Fig. 3.
A part of the spectral amplitude of
periodic pulsations in the TESS light curve figure around the |
d
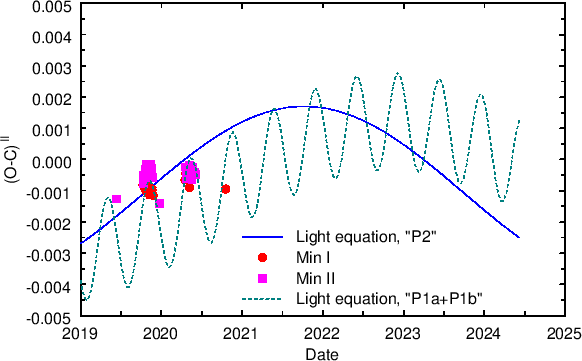
The spectral window and spectral amplitude are shown in Figure 3.
It can be seen from this plot that maxima of these functions are
at different frequencies, and they are shifted by at least a half
of their widths, so the pulsations found in the light curve seem
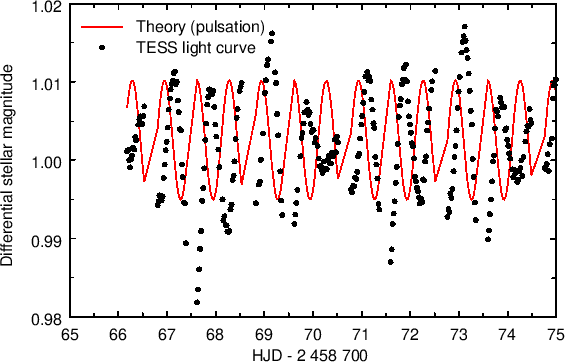
to be real. Figure 4 shows the
d pulsation
during 10 days between JD 2458766 and 2458777.
3. Photometric elements
Table 2 presents orbital elements and parameters of the system
found from the light curves obtained from ground-based
observations in 2009 at the Tien Shan observatory and from
observations by TESS. We use the following designations: 
and
are relative radii of the components in units of the
semi-major axis of the system; 
is the orbital inclination;
, eccentricity of the orbit; 
, the periastron longitude
of the orbit of the primary; 
and 
are luminosities of
the components in units of the binary's total luminosity; 
is
the third light in the same units; 
and 
are limb
darkening coefficients; is the standard deviation. The
model and method of minimization were described by Khaliullina &
Khaliullin (1984), Kozyreva & Zakharov (2001). The parameters
were calculated in a free search except the limb darkening
coefficients that had been fixed at values taken from van Hamme
(1993) taking into account the spectral types of components and
the band of observations.
The model of spherical stars (that was used in the computer program) describes detached systems adequately. The binary under investigation, with relative radii of stars 0.2 and 0.15, is close to contact. This is a potential explanation of the difference of radii of stars that can be found between the "ground-based" and "space" observations.
4. Times of minima
Tables 3 and 4 present times of minima of V957 Cep, from
ground-based observations (from the literature and from our data)
as well as from space observations. Minima from our ground-based
light curves and from TESS space light curves were calculated
using the program by Kozyreva & Zakharov (2001) jointly with the
photometric elements by minimization of differences between the
theoretical and observed light curves. In Table 3,
(O-C)
are residuals from light elements (2) (see
Section 5) and (O-C)
, from light elements (4). In
Table 4, (O-C) are residuals from light elements (3)
(see Section 5) and (O-C), from light elements (5).
Due to features of the TESS duty cycle, an individual light curve within a particular minimum contains insufficient number of points, therefore the time of minimum can be calculated with a high uncertainty. Therefore, we were forced to combine two light curves in neighboring minima. The time of the minimum in Tables 3 and 4 belongs to the earliest of the two neighboring minima.
| HJD-2400000 | (O-C) |
(O-C) |
Reference |
| 51504.6660 | 0.0016 | 0.0020 | Otero et al., 2006 |
| 54710.4925 | -0.0021 | -0.0004 | Brat et al., 2008 |
| 55076.4176 | -0.0032 | -0.0010 | Brat et al., 2008 |
| 55122.1580 | -0.0036 | 0.0007 | Kozyreva & Kusakin, 2014 |
| 55806.2827 | -0.0021 | -0.0017 | Kozyreva & Kusakin, 2014 |
| 56536.1511 | 0.0021 | -0.0003 | Kozyreva & Kusakin, 2014 |
| 57558.3524 | -0.0045 | -0.0023 | ground |
| 58123.1541 | -0.0027 | 0.0016 | ground |
| 58125.1421 | -0.0034 | 0.0009 | ground |
| 59141.3869 | -0.0009 | -0.0010 | ground |
| 58767.5053 | -0.0008 | 0.0006 | TESS |
| 58771.4828 | -0.0007 | 0.0005 | TESS |
| 58775.4601 | -0.0009 | 0.0002 | TESS |
| 58781.4262 | -0.0010 | -0.0001 | TESS |
| 58785.4039 | -0.0008 | -0.0000 | TESS |
| 58793.3587 | -0.0009 | -0.0002 | TESS |
| 58797.3359 | -0.0011 | -0.0005 | TESS |
| 58805.2911 | -0.0009 | -0.0002 | TESS |
| 58809.2685 | -0.0009 | -0.0002 | TESS |
| 58813.2458 | -0.0012 | -0.0003 | TESS |
| 58958.4238 | -0.0007 | -0.0001 | TESS |
| 58962.4014 | -0.0005 | -0.0001 | TESS |
| 58966.3789 | -0.0005 | -0.0002 | TESS |
| 58970.3562 | -0.0006 | -0.0004 | TESS |
| 58974.3339 | -0.0004 | -0.0003 | TESS |
| 58978.3109 | -0.0009 | -0.0009 | TESS |
| 58986.2665 | -0.0002 | -0.0003 | TESS |
| 58990.2436 | -0.0005 | -0.0005 | TESS |
| 58994.2211 | -0.0005 | -0.0005 | TESS |
| 59000.1874 | -0.0004 | -0.0003 | TESS |
| 59006.1536 | -0.0004 | -0.0001 | TESS |
| HJD-2400000 |
 |
 |
Reference |
| 54741.4657 | -0.0044 | -0.0012 | Brat et al., 2008 |
| 55089.4948 | -0.0042 | -0.0013 | Brat et al., 2008 |
| 55093.4725 | -0.0039 | -0.0008 | Brat et al., 2008 |
| 55113.3595 | -0.0043 | -0.0002 | ground |
| 55121.3147 | -0.0040 | 0.0003 | Kozyreva & Kusakin, 2014 |
| 55819.3656 | 0.0005 | 0.0013 | Kozyreva & Kusakin, 2014 |
| 56533.3253 | 0.0042 | 0.0018 | Kozyreva & Kusakin, 2014 |
| 57557.5182 | -0.0014 | 0.0008 | ground |
| 58649.3333 | -0.0013 | 0.0011 | ground |
| 58842.2403 | -0.0014 | 0.0007 | ground |
| 58766.6693 | -0.0005 | 0.0009 | TESS |
| 58770.6465 | -0.0008 | 0.0005 | TESS |
| 58780.5907 | -0.0003 | 0.0006 | TESS |
| 58784.5683 | -0.0002 | 0.0006 | TESS |
| 58792.5228 | -0.0006 | 0.0001 | TESS |
| 58796.5004 | -0.0004 | 0.0002 | TESS |
| 58804.4556 | -0.0002 | 0.0006 | TESS |
| 58808.4330 | -0.0003 | 0.0005 | TESS |
| 58812.4101 | -0.0006 | 0.0003 | TESS |
| 58957.5880 | -0.0002 | 0.0003 | TESS |
| 58961.5652 | -0.0005 | -0.0001 | TESS |
| 58965.5426 | -0.0006 | -0.0004 | TESS |
| 58969.5202 | -0.0005 | -0.0003 | TESS |
| 58973.4978 | -0.0004 | -0.0003 | TESS |
| 58977.4754 | -0.0002 | -0.0002 | TESS |
| 58985.4302 | -0.0004 | -0.0004 | TESS |
| 58989.4073 | -0.0007 | -0.0007 | TESS |
| 58993.3852 | -0.0003 | -0.0003 | TESS |
| 58999.3513 | -0.0004 | -0.0003 | TESS |
| 59005.3174 | -0.0005 | -0.0002 | TESS |
5. Apsidal motion
During recent nights of observations, was close to
. In such a configuration (similarly to the case of
), a precise determination of the
eccentricity and the primary's periastron longitude
was very difficult, because widths of minima were very close to
each other, the phase shift rate of the secondary minimum was
strongly reduced (the orbit practically was "stopped" for the
observer). In this configuration, the apsidal motion rate,
calculated as the linear change of with fixed
eccentricity, has a very high uncertainty, so this method was not
apt for this case.
An analysis that included all times of minima permitted a more
precise determination of the apsidal motion rate for V975 Cep in
such orientation of its orbit with respect to the observer.
Minimizing residuals between observed and calculated times of the
primary and secondary minima, O-C, we found the following
parameters: 
, 
, 
, 
, 
.
Ephemerides (2) and (3) were used as calculated (C) times (
is
the number of cycles from the initial epoch):
Ephemerides that include the apsidal motion of the system are the following:
The results are collected in Table 5 and shown in Fig. 5. The
figure presents residuals (O-C) and theoretical curves that
reflect sinusoidal variations of the times of minima due to
apsidal motion. For clarity, we show the point where the primary's
periastron longitude was 
(close to the year 1960)
and the phase of the secondary minimum was 0.5.
 |
Fig. 5.
(O-C) |
6. Light-time effect
(all models)
 |
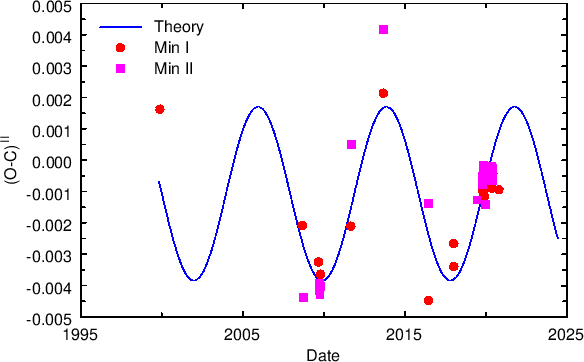Fig. 6.
(O-C) |
Figures 6 and 7 show (O-C) for minima of V957 Cep,
where O is the observed time and C, the calculated time (equations
(4) and (5)). The changes of these residuals (Fig. 6) for primary
and secondary minima are synchronous, so the system exhibits the
light-time effect and thus we may expect the presence of one or
several additional bodies. Parameters of the light-time effect are
presented in Table 6. Figure 7 shows the recent part of
observations, it contains two curves: "P1a+P1b" describes the
sum of two periodical variations "P1a" and "P1b", "P2"
describes the light-time effect as a single variation.
Attempts to compute parameters of the orbit of the third body by
minimization of the light-time effect, as it was done by Kozyreva
& Khaliullin (1999), show a period about
and eccentricity 
(this solution is not shown in the figures). The duration of
observations of this star from 2009 to 2021 is insufficient to
reliably confirm this orbit. A lot of times of minima obtained by
TESS only weakly influence the accuracy of the determination of
parameters of the third body's orbit, because these minima
compactly lie within a short time interval. The current set of
observations makes it possible to more or less reliably estimate
the period and amplitude of the light-time effect. To find these
parameters, we used the PERDET code. Figure 8 shows the power
spectrum of (O-C). The analysis of
(O-C) residuals shows that the sum of two periods,
and
, satisfies the light-time
effect with minimal 
(marks "1" and "2" in
Fig. 8). The contribution of the 
period is most important to
reduce the mean-square scatter between the theoretical and
observed times, (O-C)
(
, see Table 6). If the
process with only one period is considered, then the best
corresponds to the light-time effect with the
period
and amplitude
.
 |
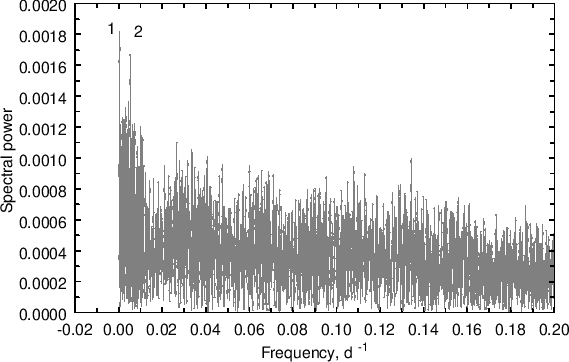Fig. 8.
Spectral power of variations of the
(O-C) |
and
.
7. Conclusions
Using observations by the TESS satellite, we found pulsations of
the V957 Cep brightness with the period 0664 and amplitude
. We also derived new photometric elements of the
system based on our light curve (2009-2019) and on the light
curve by TESS (2019-2020), and re-estimated the apsidal motion
rate
yr.
Analyzing all times of minima, we found evidence for the presence of light-time effect in the system. It can be caused by the gravitational influence of an additional body (or even additional bodies), gravitationally bound with the binary. Our calculations show a very high eccentricity of the third body (0.8), but this result needs confirmation with future observations, because a longer set of observations is required. Currently we can only indicate most probable values for the amplitude of the light-time effect (4 minutes) and for its period (8 years). It is important to precisely find spectral types of both components of the binary and to increase the number of observed times of minima in order to derive orbital parameters of the third body (probably also using the radial velocity curve that has not been obtained yet).
Acknowledgments
This research has made use of the SIMBAD database (operated at CDS, Strasbourg, France) and of NASA's Astrophysics Data System. Some of the data presented in this paper were obtained from the Mikulski Archive for Space Telescopes (MAST). This research is funded by the Science Committee of the Ministry of Education and Science of the Republic of Kazakhstan (Grant No. AP09259383).
References:
Brát, L., Šmelcer, L., Kucáková, H., et al., 2008, Open European Journal on Variable stars, 94, 1
Breger, M., 1990, Delta Scuti Star Newsletter, 2, 21
Khaliullina, A.I., Khaliullin, K.F., 1984, Soviet Astronomy, 28, 228
Kozyreva, V.S., Khaliullin, Kh.F., 1999, Astronomy Reports, 43, 679
Kozyreva, V.S., Kusakin, A.V., Menke, J., 2012, Inform. Bull. Var. Stars, No. 6020
Kozyreva, V.S., Kusakin, A.V., 2014, Astrophysics, 57, 221
Kozyreva, V.S., Zakharov, A.I., 2001, Astronomy Letters, 27, 712
Otero, S.A., Wils, R., Hoogeveen, G., Dubovsky, P.A., 2006, Inform. Bull. Var. Stars, No. 5681
Stellingwerf, R.F., 1978, Astrophys. J., 224, 953.
Sterne, T.E., 1939, Monthly Notices Roy. Astron. Soc., 99, 451
van Hamme, W., 1993, Astron. J., 106, 2096
Wozniak, P. R., Vestrand, W. T., Akerlof, C. W., et al., 2004, Astron. J., 127, 2436