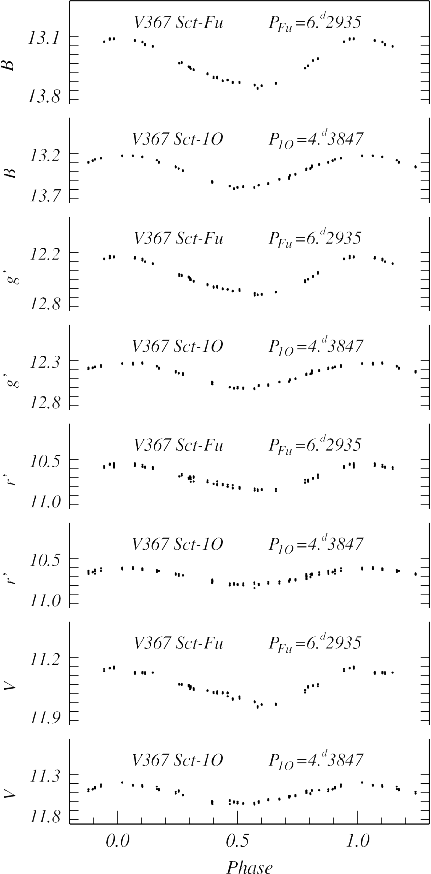|
Peremennye Zvezdy (Variable Stars) 44, No. 3, 2024 Received 28 April; accepted 24 May. |
Article in PDF |
|
DOI: 10.24412/2221-0474-2024-44-28-41
|
Search for Evolutionary Period Changes in the Double-mode Cepheid V367 Sct
L. N. Berdnikov[1], A. A. Belinskii[1], A. K. Dambis [1], E. N. Pastukhova[2], M. A. Burlak[1], N. P. Ikonnikova[1], E. O. Mishin[1], N. I. Shatskii[1]
- Sternberg Astronomical Institute, Moscow State University,
Universitetskij pr. 13, Moscow 119992, Russia;
leonid.berdnikov@gmail.com
- Institute of Astronomy, Russian Academy of Sciences, ul. Pyatnitskaya 48, Moscow, 119017, Russia
|
For both periods of the bimodal Cepheid V367 Sct, O-C
diagrams spanning a 113-year time interval are constructed. The
O-C diagrams have the form of parabolas, allowing quadratic
ephemerides and evolutionary period change rates to be determined
for the first time,
|
It follows from the computations performed by Eggenberger et al.
(2021), Nguyen et al. (2022), and Yusof et al. (2022) that the
evolutionary tracks of short-period Cepheids with periods shorter
than 5 days (masses less than ![]() ) either have no blue
loop after the first crossing of the instability strip or this
loop does not reach the instability strip, which means that the
second and third crossings do not occur. This means that all
short-period Cepheids are observed at the first crossing.
) either have no blue
loop after the first crossing of the instability strip or this
loop does not reach the instability strip, which means that the
second and third crossings do not occur. This means that all
short-period Cepheids are observed at the first crossing.
According to the theory, the periods of Cepheids during the first crossing increase so rapidly that the O-C diagrams should be parabolas with steep upward branches. However, no steep parabolas, i.e. rapid evolutionary period changes, have been found in the O-C diagrams for 41 short-period Cepheids (hereafter referred to simply as Cepheids) studied over a time interval of more than a hundred years (Csoernyei et al., 2022). The O-C diagrams of such Cepheids look like small-amplitude semi-regular oscillations, which are sometimes superimposed onto a slight trend; if this trend is interpreted as a result of evolutionary period changes, then the rate of these changes formally corresponds to the second or third crossing of the instability strip (Turner et al., 2006).
As of now, rapid evolutionary period changes have been found only
for three Cepheids. These are two normal Cepheids, V1033 Cyg and
OGLE-LMC-CEP-2132 with the periods of
![]() and
and
![]() , respectively (Berdnikov et al., 2019, 2023) and one
bimodal Cepheid, V371 Per, pulsating both in the fundamental tone
and first overtone with the periods of
, respectively (Berdnikov et al., 2019, 2023) and one
bimodal Cepheid, V371 Per, pulsating both in the fundamental tone
and first overtone with the periods of
![]() and
and
![]() , respectively (Berdnikov et al.,
2023). In order to understand how these three Cepheids differ from
the others, first of all, it is necessary to increase the sample
size, that is, to investigate period changes in unexplored
Cepheids.
, respectively (Berdnikov et al.,
2023). In order to understand how these three Cepheids differ from
the others, first of all, it is necessary to increase the sample
size, that is, to investigate period changes in unexplored
Cepheids.
One of such objects is the bimodal Cepheid V367 Sct, and the aim of this study is to search for evolutionary changes of its pulsation periods.
1. LIGHT CURVES FOR BOTH OSCILLATIONS OF V367 Sct
From July 16 to October 10, 2021, we acquired 379 ![]() -band
CCD frames for the bimodal Cepheid V367 Sct with the 60-cm
telescope of the Caucasian Mountain Observatory of Sternberg
Astronomical Institute of M.V.Lomonosov Moscow State University
(Berdnikov et al., 2021). The method of constructing light curves
(Fig. 1) for the fundamental mode (
-band
CCD frames for the bimodal Cepheid V367 Sct with the 60-cm
telescope of the Caucasian Mountain Observatory of Sternberg
Astronomical Institute of M.V.Lomonosov Moscow State University
(Berdnikov et al., 2021). The method of constructing light curves
(Fig. 1) for the fundamental mode (
![]() ) and
the first overtone (
) and
the first overtone (
![]() ) is described by
Berdnikov et al. (2021).
) is described by
Berdnikov et al. (2021).
Table 1 lists the ![]() -band light-curve parameters for both
oscillations of V367 Sct: magnitude at maximum light, amplitude,
and intensity-mean magnitude. Table 2 lists the Fourier
coefficients (for the cosine expansion). The Fourier coefficients
for
-band light-curve parameters for both
oscillations of V367 Sct: magnitude at maximum light, amplitude,
and intensity-mean magnitude. Table 2 lists the Fourier
coefficients (for the cosine expansion). The Fourier coefficients
for ![]() fall within the domains of classical (DCEP)
Cepheids and those for
fall within the domains of classical (DCEP)
Cepheids and those for ![]() , within the domain of
low-amplitude Cepheids (DCEPS), which pulsate in the first
overtone (Udalski et al., 2018).
, within the domain of
low-amplitude Cepheids (DCEPS), which pulsate in the first
overtone (Udalski et al., 2018).
2. TECHNIQUE OF STUDYING PERIOD CHANGES AND OBSERVATIONAL DATA USED
We investigate changes in Cepheid pulsation periods using the standard technique of the analysis of O-C diagram. The O-C residuals can be most accurately determined using the Hertzsprung method (Hertzsprung, 1919) whose computer implementation is described by Berdnikov (1992f). We use the method described by Lombard & Koen (1993) to confirm the reality of the period changes found.
To study the periods of V367 Sct, we compiled published photographic, photoelectric, CCD observations. We supplemented these data with our own eye estimates of the star's magnitudes on photographic plates of the Sternberg Astronomical Institute plate archive and with ASAS-3 (Pojmanski, 2002) and ASAS-SN (Jayasinghe et al., 2019) survey photometry.
Table 3 summarizes information about the number of observations used. These observations span a 113-year time interval.
3. RESULTS AND DISCUSSION
We use the method described by Berdnikov et al. (2021) to extract
the light curves corresponding to the fundamental mode and first
overtone from observations of V367 Sct (Fig. 1). We computed
seasonal light curves based on the data obtained; Table 4
summarizes the results of their analysis performed using the
Hertzsprung method. The first and second columns of this table
give the times of maximum light and their errors; the third column
gives the type of observations; the fourth and fifth columns
present the epoch number ![]() and the O-C residual, respectively;
the sixth and seventh columns are the number of observations
and the O-C residual, respectively;
the sixth and seventh columns are the number of observations ![]() and the source of data, respectively. The data from Table 4 are
shown in the O-C diagrams (Figs. 2 and 3 for the fundamental
mode and first overtone, respectively) as squares for photographic
observations and small filled circles for other observations, with
vertical error bars of O-C residuals.
and the source of data, respectively. The data from Table 4 are
shown in the O-C diagrams (Figs. 2 and 3 for the fundamental
mode and first overtone, respectively) as squares for photographic
observations and small filled circles for other observations, with
vertical error bars of O-C residuals.
The O-C diagrams have the form of parabolas. Based on the times of maximum light from Table 4, we inferred the quadratic ephemerides listed in Table 5, which we use to draw the parabolas in the top panels of Figs. 2 and`3. The bottom panels of these figures show the residuals from these parabolas. We use the linear parts of these ephemerides to compute the O-C residuals in the fifth column of Table 4.
We use the data from Table 4 to compute the differences between
the times of maximum light in the ![]() band (and also in
photographic, pg, magnitudes whose photometric system is close to
that of the
band (and also in
photographic, pg, magnitudes whose photometric system is close to
that of the ![]() band), and
band), and ![]() and
and ![]() bands relative to the
bands relative to the ![]() band; the corresponding corrections are listed in Table 6. We used
these corrections when drawing Figs. 2 and 3 and computing the
ephemerides (Table 5), which therefore apply to the
band; the corresponding corrections are listed in Table 6. We used
these corrections when drawing Figs. 2 and 3 and computing the
ephemerides (Table 5), which therefore apply to the ![]() -band
variations.
-band
variations.
We use the method published by Lombard and Koen (1993) to confirm
the reality of the changes in the pulsation period. To this end,
we compute the differences
![]() of
consecutive O-C residuals from Table 4,
of
consecutive O-C residuals from Table 4,
![]() , and plot
the dependences of
, and plot
the dependences of
![]() on
on
![]() for both oscillations of V367 Sct
(Figs. 4 and 5). The
for both oscillations of V367 Sct
(Figs. 4 and 5). The ![]() values, which have the meaning of
average period in the epoch interval
values, which have the meaning of
average period in the epoch interval ![]() -
-![]() ,
correspond to the behavior of the O-C residuals in Figs. 2 and 3,
and hence the period increases found are real.
,
correspond to the behavior of the O-C residuals in Figs. 2 and 3,
and hence the period increases found are real.
The quadratic terms (Table 5) allow us to compute the rates of evolutionary period increase for the fundamental tone and first overtone of V367 Sct, which are listed in the fifth column of Table 5. These period increase rates are consistent with theoretical computations for the third crossing of the instability strip (Turner et al., 2006; Fadeyev, 2014).
4. Conclusions
To study the period changes of the bimodal Cepheid V367 Sct, we
acquired 379 CCD frames in ![]() filters with the 60-cm
telescope of Caucasian Mountain Observatory and made 282 magnitude
estimates on photographic plates of Sternberg Astronomical
Institute (Moscow); in addition, we compiled 4329 published
photometric observations. We used the Hertzsprung method to
determine 203 times of maximum light for both oscillations, which
allowed us to construct O-C diagrams spanning a 113-year time
interval. The O-C diagrams have the form of parabolas, which
allowed us, for the first time, to determine the quadratic
ephemerides and to compute the rates of evolutionary period
changes:
filters with the 60-cm
telescope of Caucasian Mountain Observatory and made 282 magnitude
estimates on photographic plates of Sternberg Astronomical
Institute (Moscow); in addition, we compiled 4329 published
photometric observations. We used the Hertzsprung method to
determine 203 times of maximum light for both oscillations, which
allowed us to construct O-C diagrams spanning a 113-year time
interval. The O-C diagrams have the form of parabolas, which
allowed us, for the first time, to determine the quadratic
ephemerides and to compute the rates of evolutionary period
changes:
![]() s/year and
s/year and
![]() s/yr for the fundamental
tone and the first overtone of V367 Sct, respectively, which is
consistent with the theoretical results for the third crossing of
the instability strip. The pulsation stability test proposed by
Lombard & Koen (1993) confirmed that the increase of periods was
real.
s/yr for the fundamental
tone and the first overtone of V367 Sct, respectively, which is
consistent with the theoretical results for the third crossing of
the instability strip. The pulsation stability test proposed by
Lombard & Koen (1993) confirmed that the increase of periods was
real.
Acknowledgments
This study was made using equipment acquired within the framework of the Development Program of M.V. Lomonosov Moscow State University.References:
Arellano Ferro, A., Rojo Arellano, E., Gonzáles-Bedolla, S., & Rosenzweig, P., 1998, Astrophys. J. Suppl. Ser., 117, 167
Berdnikov, L. N., 1986, Variable Stars, 22, 369
Berdnikov, L. N., 1992a, Astron. & Astrophys. Trans., 2, 1
Berdnikov, L.N., 1992b, Astron. & Astrophys. Trans., 2, 43
Berdnikov, L.N., 1992c, Astron. & Astrophys. Trans., 2, 107
Berdnikov, L.N., 1992d, Astron. & Astrophys. Trans., 2, 157
Berdnikov, L. N., 1992e, Sov. Astron. Letters, 18, 130
Berdnikov, L. N., 1992f, Sov. Astron. Letters, 18, 207
Berdnikov, L. N. & Caldwell, J. A. R., 2001, J. Astron. Data, 7, No. 3
Berdnikov, L. N. & Ibragimov, M. A., 1994a, Astron. & Astrophys. Trans., 5, 317
Berdnikov, L. N. & Ibragimov, M. A., 1994b, Inform. Bull. Var. Stars, No. 3991, 1
Berdnikov, L. N. & Turner, D. G., 1995a, Astron. Lett., 21, 534
Berdnikov, L. N. & Turner, D. G., 1995b, Astron. Lett., 21, 717
Berdnikov, L. N. & Turner, D. G., 1998a, Astron. & Astrophys. Trans., 16, 205
Berdnikov, L. N. & Turner, D. G., 1998b, Astron. & Astrophys. Trans., 16, 291
Berdnikov, L. N. & Turner, D. G., 2000, Astron. & Astrophys. Trans., 18, 679
Berdnikov, L. N. & Turner, D. G., 2001, Astron. & Astrophys. Trans., 19, 689
Berdnikov, L. N., Kniazev, A.`Yu., Dambis, A. K., & Kravtsov, V. V., 2019, Variable Stars, 39, No. 1
Berdnikov, L. N., Belinskij, A. A., Pastukhova, E. N., et al., 2021, Variable Stars, 41, No. 2, 1
Berdnikov, L. N., Belinskij, A. A., Pastukhova, E. N., et al., 2023, Astron. Letters, 49, in press
Berdnikov, L. N., Belinskij, A. A., Shatskij, N. I., et al., 2020, Astron. Reports, 64, 310
Berdnikov, L. N., Ignatova, V. V., & Vozyakova, O. V., 1997, Astron. & Astrophys. Trans., 14, 237
Berdnikov, L. N., Ignatova, V. V., & Vozyakova, O. V., 1998, Astron. & Astrophys. Trans., 17, 87
Berdnikov, L. N., Pastukhova, E. N., Kovtyukh, V. V., et al., 2019, Astron. Letters, 45, 229
Berdnikov, L. N., Vozyakova, O. V., & Ibragimov, M. A., 1995, Inform. Bull. Var. Stars, No. 4141, 1
Csoernyei, G., Szabados, L., Molnar, L., et al., 2022, Mon. Notices Roy. Astron. Soc., 511, 2155
Dean, J. F., 1977, Mon. Not. Astron. Soc. South Africa, 36, 3
Efremov, Yu. N. & Kholopov, P. N., 1975, Variable Stars, 20, 133
Eggenberger, P., Ekstrom, S., Georgy, C., et al., 2021, Astron. & Astrophys., 652, A137
Fadeyev, Yu. A., 2014, Astron. Letters, 40, 301
Hertzsprung, E., 1919, Astron. Nachr., 208, 51
Ignatova, V. V. & Vozyakova, O. V., 2000, Astron. & Astrophys. Trans., 19, 133
Jayasinghe, T., Kochanek, C. S., Stanek, K. Z., et al., 2019, Mon. Notices Roy. Astron. Soc., 485, 961
Lombard, F. & Koen, Ch., 1993, Mon. Notices Roy. Astron. Soc., 263, 309
Madore, B. F., Stobie, B. F., & van den Bergh, S., 1978, Mon. Notices Roy. Astron. Soc., 183, 13
Madore, B. F. & van den Bergh, S., 1975, Astrophys. J., 197, 55
Moffett, T. J. & Barnes, T. G., 1984, Astrophys. J. Suppl. Ser., 55, 389
Nguyen, C. T., Costa, G., Girardi, L. et al., 2022, arXiv:2207.08642v1
Pojmanski, G., 2002, Acta Astron., 52, 397
Roslund, C. & Pretorius, W., 1962, Arkiv Astron., 3, 201
Turner, D. G., Abdel-Sabour Abdel-Latif, M., & Berdnikov, L. N., 2006, Publ. Astron. Soc. Pacif., 118, 410
Udalski, A., Soszynski, I., Pietrukowicz, P., et al., 2018, Acta Astron., 68, 315
Yusof, N., Hirschi, R., Eggenberger, P., et al., 2022, Mon. Notices Roy. Astron. Soc., 511, 2814
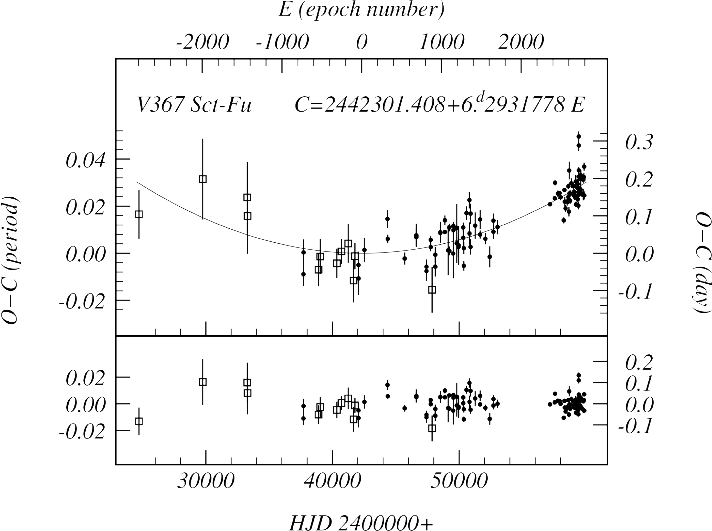 |
Fig. 2. The O-C diagram for V367 Sct for linear (top panel) and quadratic (bottom panel) ephemeris of the fundamental tone (Table 5). The curve shows the parabola corresponding to the ephemeris (Table 5). Symbols are explained in the text. |
 |
Fig. 3. The O-C diagram for V367 Sct for linear (top panel) and quadratic (bottom panel) ephemeris of the first overtone (Table 5). The curve shows the parabola corresponding to the ephemeris (Table 5). Symbols are explained in the text. |
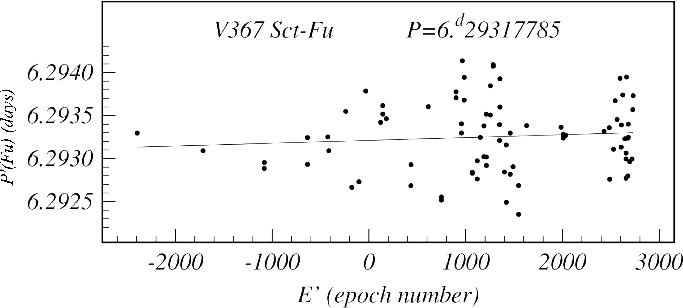 |
Fig. 4.
The dependence of
|
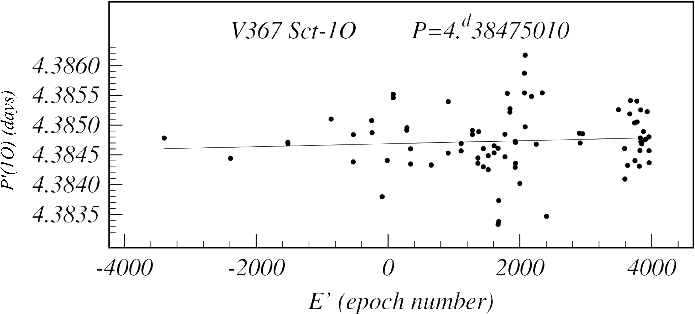 |
Fig. 5.
The dependence of
|
Table 1. Parameters of ![]() -,
-, ![]() -,
-, ![]() -, and
-, and ![]() -band
light curves of both oscillations of V367 Sct
-band
light curves of both oscillations of V367 Sct
| Oscillation mode | Filter | Magnitude at | Amplitude | Intensity-mean |
| maximum light | magnitude | |||
| Fundamental mode | |
13.117 | 0.531 | 13.385 |
| Fundamental mode | |
11.433 | 0.379 | 11.618 |
| Fundamental mode | |
12.292 | 0.438 | 12.505 |
| Fundamental mode | |
10.551 | 0.303 | 10.704 |
| First overtone | |
13.225 | 0.352 | 13.385 |
| First overtone | |
11.486 | 0.244 | 11.618 |
| First overtone | |
12.346 | 0.288 | 12.505 |
| First overtone | |
10.607 | 0.201 | 10.704 |
Table 2. Fourier coefficients
(cosine expansion) of the ![]() -,
-, ![]() -,
-, ![]() -, and
-, and ![]() -band light
curves of the fundamental mode (
-band light
curves of the fundamental mode (
![]() ) and
first overtone (
) and
first overtone (
![]() ) of V367 Sct.
) of V367 Sct.
| Oscillation | Filter | |
|
|
|
|
|
|
| mode | Error | Error | Error | Error | Error | Error | ||
| Fundamental mode | |
0.11100 | 0.02040 | 0.01350 | 4.27050 | 2.29911 | 4.09857 | |
| |
|
|
|
|
|
|||
| Fundamental mode | 0.15596 | 0.03487 | 0.01320 | 4.48043 | 2.36722 | 6.24081 | ||
| |
|
|
|
|
|
|||
| Fundamental mode | 0.16781 | 0.00839 | 0.02262 | 4.22781 | 1.72122 | 0.12706 | ||
| |
|
|
|
|
|
|||
| First overtone | |
0.03281 | 0.00873 | 0.00002 | 5.40537 | 3.85542 | 2.34633 | |
| |
|
|
|
|
|
|||
| First overtone | |
0.12838 | 0.00423 | 0.00188 | 3.74491 | 1.48809 | 1.90353 | |
| |
|
|
|
|
|
|||
| First overtone | 0.08753 | 0.00652 | 0.00015 | 3.66465 | 3.60980 | 2.29634 | ||
| |
|
|
|
|
|
|||
| First overtone | 0.03161 | 0.01210 | 0.02313 | 0.61989 | 2.03573 | 3.30126 | ||
| |
|
|
|
|
|
|||
Table 3. Observational data for V367 Sct.
| Data source | Number of | Type of | JD interval |
| observations | observations | ||
| Caucasian Mountain Observatory (this paper) | 379 | CCD, |
2459403-2459438 |
| Sternberg Astronomical Institute (this paper) | 282 | Photographic, pg | 2432826-2448179 |
| Published | 314 | Photographic, pg | 2418529-2441926 |
| Published | 860 | Photoelectric, |
2437549-2453118 |
| ASAS-3 | 223 | CCD, |
2452135-2453228 |
| ASAS-SN | 2932 | CCD, |
2457061-2460044 |
Table 4. Times of maximum light for V367 Sct
| Maximum, HJD | Error, days | Filter | E | O - C, days | N | Reference |
| V367 Sct-Fu | ||||||
| 2424718.3580 | 0.0656 | pg | |
|
58 | Efremov, Kholopov (1975) |
| 2429752.9944 | 0.1084 | pg | |
|
35 | Efremov, Kholopov (1975) |
| 2433277.1252 | 0.0949 | pg | |
|
22 | Sternberg Astronomical Institute |
| 2433295.9548 | 0.1011 | pg | |
|
22 | Efremov, Kholopov (1975) |
| 2437707.3317 | 0.0315 | |
|
|
25 | Roslund, Pretorius (1962) |
| 2437713.6682 | 0.0351 | |
|
|
24 | Roslund, Pretorius (1962) |
| 2438896.7396 | 0.0446 | pg | |
|
57 | Efremov, Kholopov (1975) |
| 2439035.2246 | 0.0469 | pg | |
|
78 | Sternberg Astronomical Institute |
| 2440344.1875 | 0.0396 | pg | |
|
75 | Efremov, Kholopov (1975) |
| 2440709.2236 | 0.0334 | pg | |
|
71 | Sternberg Astronomical Institute |
| 2441244.1650 | 0.0518 | pg | |
|
45 | Efremov, Kholopov (1975) |
| 2441665.7087 | 0.0586 | pg | |
|
35 | Efremov, Kholopov (1975) |
| 2441772.7581 | 0.0353 | pg | |
|
81 | Sternberg Astronomical Institute |
| 2442055.9425 | 0.0362 | |
|
|
37 | Madore, van den Bergh (1975) |
| 2442062.1848 | 0.0447 | |
|
|
36 | Madore, van den Bergh (1975) |
| 2442515.3697 | 0.0322 | |
|
|
54 | Madore et al. (1978) |
| 2444346.7675 | 0.0243 | |
|
|
124 | Moffett, Barnes (1984) |
| 2444365.6087 | 0.0118 | |
|
|
44 | Moffett, Barnes (1984) |
| 2445699.7099 | 0.0165 | |
|
|
50 | Berdnikov (1986) |
| 2446618.5607 | 0.0315 | |
|
|
27 | Berdnikov (1992a) |
| 2446618.5714 | 0.0234 | |
|
|
27 | Berdnikov (1992a) |
| 2447417.7103 | 0.0196 | |
|
|
30 | Berdnikov (1992b) |
| 2447417.7136 | 0.0264 | |
|
|
30 | Berdnikov (1992b) |
| 2447757.5945 | 0.0118 | |
|
|
41 | Berdnikov (1992c) |
| 2447757.6289 | 0.0101 | |
|
|
41 | Berdnikov (1992c) |
| 2447864.4644 | 0.0617 | pg | |
|
30 | Sternberg Astronomical Institute |
| 2448116.2532 | 0.0267 | |
|
|
38 | Berdnikov (1992d) |
| 2448116.3004 | 0.0212 | |
|
|
19 | Berdnikov (1992d) |
| 2448512.8153 | 0.0284 | |
|
|
31 | Berdnikov (1992e) |
| 2448512.8284 | 0.0137 | |
|
|
62 | Berdnikov (1992e) |
| 2448877.8204 | 0.0052 | |
|
|
824 | Berdnikov, Ibragimov (1994a) |
| 2448877.8671 | 0.0130 | |
|
|
83 | Berdnikov, Ibragimov (1994a) |
| 2449129.5138 | 0.0670 | |
|
|
45 | Arellano Ferro et al. (1998) |
| 2449223.9098 | 0.0123 | |
|
|
50 | Berdnikov, Ibragimov (1994b) |
| 2449223.9571 | 0.0108 | |
|
|
98 | Berdnikov, Ibragimov (1994b) |
| 2449538.5617 | 0.0663 | |
|
|
10 | Berdnikov, Turner (1995a) |
| 2449544.9139 | 0.0098 | |
|
|
164 | Berdnikov et al. (1995) |
| 2449551.2100 | 0.0122 | |
|
|
83 | Berdnikov et al. (1995) |
| 2449815.4734 | 0.0527 | |
|
|
15 | Berdnikov, Turner (1995b) |
| 2449815.5299 | 0.0644 | |
|
|
15 | Berdnikov, Turner (1995b) |
| 2449947.6206 | 0.0271 | |
|
|
56 | Berdnikov et al. (1997) |
| 2449960.2264 | 0.0525 | |
|
|
23 | Berdnikov et al. (1997) |
| 2450325.2512 | 0.0224 | |
|
|
33 | Berdnikov et al. (1998) |
| 2450325.2634 | 0.0136 | |
|
|
133 | Berdnikov et al. (1998) |
| 2450369.2132 | 0.0132 | |
|
|
28 | Berdnikov, Turner (1998a) |
| 2450369.2757 | 0.0162 | |
|
|
28 | Berdnikov, Turner (1998a) |
| 2450577.0441 | 0.0185 | |
|
|
35 | Berdnikov, Turner (1998b) |
| 2450809.9265 | 0.0225 | |
|
|
45 | Ignatova, Vozyakova (2000) |
| 2450816.1151 | 0.0302 | |
|
|
44 | Ignatova, Vozyakova (2000) |
| 2450904.1837 | 0.0210 | |
|
|
94 | Berdnikov, Turner (2000) |
| 2450904.2879 | 0.0271 | |
|
|
48 | Berdnikov, Turner (2000) |
| 2451269.2451 | 0.0352 | |
|
|
23 | Berdnikov, Turner (2001) |
| 2451653.1203 | 0.0108 | |
|
|
21 | Berdnikov, Caldwell (2001) |
| 2451653.1456 | 0.0256 | |
|
|
42 | Berdnikov, Caldwell (2001) |
| 2452074.7521 | 0.0155 | |
|
|
54 | ASAS |
| 2452414.5353 | 0.0282 | |
|
|
50 | ASAS |
| Table 4. Continued | ||||||
| Maximum, HJD | Error, days | Filter | E | O - C, days | N | Reference |
| V367 Sct-Fu | ||||||
| 2452704.0885 | 0.0190 | |
|
|
66 | Berdnikov et al. (2019) |
| 2452704.1182 | 0.0191 | |
|
|
48 | ASAS |
| 2453031.3463 | 0.0198 | |
|
|
59 | ASAS |
| 2457172.3183 | 0.0037 | |
|
|
110 | ASAS-SN |
| 2457543.6319 | 0.0035 | |
|
|
99 | ASAS-SN |
| 2457575.1390 | 0.0072 | |
|
|
60 | ASAS-SN |
| 2457795.3714 | 0.0083 | |
|
|
60 | ASAS-SN |
| 2457927.5295 | 0.0028 | |
|
|
154 | ASAS-SN |
| 2458009.3293 | 0.0083 | |
|
|
60 | ASAS-SN |
| 2458254.7019 | 0.0083 | |
|
|
62 | ASAS-SN |
| 2458311.4215 | 0.0035 | |
|
|
120 | ASAS-SN |
| 2458336.4655 | 0.0130 | |
|
|
60 | ASAS-SN |
| 2458399.3794 | 0.0093 | |
|
|
59 | ASAS-SN |
| 2458525.2606 | 0.0105 | |
|
|
55 | ASAS-SN |
| 2458613.3781 | 0.0112 | |
|
|
60 | ASAS-SN |
| 2458619.6996 | 0.0076 | |
|
|
70 | ASAS-SN |
| 2458625.9558 | 0.0110 | |
|
|
55 | ASAS-SN |
| 2458669.9773 | 0.0129 | |
|
|
74 | ASAS-SN |
| 2458695.2601 | 0.0240 | |
|
|
59 | ASAS-SN |
| 2458714.0785 | 0.0070 | |
|
|
60 | ASAS-SN |
| 2458764.4038 | 0.0078 | |
|
|
70 | ASAS-SN |
| 2458764.4232 | 0.0093 | |
|
|
54 | ASAS-SN |
| 2458865.1417 | 0.0135 | |
|
|
59 | ASAS-SN |
| 2459016.1705 | 0.0095 | |
|
|
69 | ASAS-SN |
| 2459060.1976 | 0.0094 | |
|
|
74 | ASAS-SN |
| 2459097.9658 | 0.0062 | |
|
|
70 | ASAS-SN |
| 2459135.7134 | 0.0098 | |
|
|
60 | ASAS-SN |
| 2459204.9781 | 0.0186 | |
|
|
58 | ASAS-SN |
| 2459223.7966 | 0.0075 | |
|
|
55 | ASAS-SN |
| 2459362.2602 | 0.0079 | |
|
|
69 | ASAS-SN |
| 2459362.2899 | 0.0085 | |
|
|
84 | ASAS-SN |
| 2459362.3011 | 0.0138 | |
|
|
60 | ASAS-SN |
| 2459412.5887 | 0.0106 | |
|
|
55 | ASAS-SN |
| 2459418.9106 | 0.0174 | |
|
|
60 | ASAS-SN |
| 2459425.2103 | 0.0121 | |
|
|
54 | ASAS-SN |
| 2459437.8389 | 0.0212 | |
|
|
93 | Sternberg Astronomical Institute |
| 2459438.0193 | 0.0153 | |
|
|
95 | Sternberg Astronomical Institute |
| 2459444.2310 | 0.0096 | |
|
|
91 | Sternberg Astronomical Institute |
| 2459569.9590 | 0.0125 | |
|
|
56 | ASAS-SN |
| 2459576.2545 | 0.0073 | |
|
|
70 | ASAS-SN |
| 2459595.1616 | 0.0216 | |
|
|
60 | ASAS-SN |
| 2459607.7585 | 0.0138 | |
|
|
66 | ASAS-SN |
| 2459645.4690 | 0.0089 | |
|
|
65 | ASAS-SN |
| 2459802.7950 | 0.0158 | |
|
|
57 | ASAS-SN |
| 2459809.1282 | 0.0082 | |
|
|
73 | ASAS-SN |
| 2459853.1872 | 0.0111 | |
|
|
68 | ASAS-SN |
| 2459872.0948 | 0.0111 | |
|
|
72 | ASAS-SN |
| 2459878.3109 | 0.0111 | |
|
|
61 | ASAS-SN |
| V367 Sct-1O | ||||||
| 2424465.1663 | 0.0778 | pg | |
|
53 | Efremov, Kholopov (1975) |
| 2430077.6886 | 0.0999 | pg | |
|
29 | Efremov, Kholopov (1975) |
| 2433278.3314 | 0.0630 | pg | |
|
22 | Sternberg Astronomical Institute |
| 2433295.8936 | 0.0844 | pg | |
|
22 | Efremov, Kholopov (1975) |
| 2437702.5100 | 0.0354 | |
|
|
25 | Roslund, Pretorius (1962) |
| 2437742.2970 | 0.0297 | |
|
|
20 | Roslund, Pretorius (1962) |
| 2439031.1910 | 0.0379 | pg | |
|
77 | Sternberg Astronomical Institute |
| 2439044.1764 | 0.0342 | pg | |
|
70 | Efremov, Kholopov (1975) |
| 2440649.0281 | 0.0495 | pg | |
|
71 | Efremov, Kholopov (1975) |
| 2440706.0701 | 0.0321 | pg | |
|
71 | Sternberg Astronomical Institute |
| 2441477.8078 | 0.0418 | pg | |
|
67 | Efremov, Kholopov (1975) |
| 2441771.5517 | 0.0328 | pg | |
|
81 | Sternberg Astronomical Institute |
| 2442052.0901 | 0.0361 | |
|
|
37 | Madore, van den Bergh (1975) |
| 2442056.4832 | 0.0456 | |
|
|
36 | Madore, van den Bergh (1975) |
| 2442442.3659 | 0.0771 | |
|
|
54 | Madore, van den Bergh (1975) |
| 2442468.6626 | 0.0373 | |
|
|
31 | Madore et al. (1978) |
| 2442951.1169 | 0.0722 | |
|
|
8 | Dean (1977) |
| 2442951.2034 | 0.0464 | |
|
|
8 | Dean (1977) |
| 2444358.5741 | 0.0202 | |
|
|
118 | Moffett, Barnes (1984) |
| 2444362.9616 | 0.0135 | |
|
|
44 | Moffett, Barnes (1984) |
| 2445695.7986 | 0.0172 | |
|
|
50 | Berdnikov (1986) |
| 2445695.9764 | 0.0574 | |
|
|
23 | Berdnikov (1986) |
| 2446621.1122 | 0.0283 | |
|
|
27 | Berdnikov (1992a) |
| 2446621.1396 | 0.0260 | |
|
|
27 | Berdnikov (1992a) |
| Table 4. Continued | ||||||
| Maximum, HJD | Error, days | Filter | E | O - C, days | N | Reference |
| V367 Sct-1O | ||||||
| 2447419.1307 | 0.0294 | |
|
|
30 | Berdnikov (1992b) |
| 2447419.1368 | 0.0166 | |
|
|
30 | Berdnikov (1992b) |
| 2447756.7685 | 0.0109 | |
|
|
41 | Berdnikov (1992c) |
| 2447756.7889 | 0.0118 | |
|
|
41 | Berdnikov (1992c) |
| 2447861.9297 | 0.0377 | |
|
|
30 | Sternberg Astronomical Institute |
| 2448111.9421 | 0.0270 | |
|
|
38 | Berdnikov (1992d) |
| 2448112.0003 | 0.0232 | |
|
|
19 | Berdnikov (1992d) |
| 2448510.8983 | 0.0309 | |
|
|
62 | Berdnikov (1992e) |
| 2448510.9268 | 0.0110 | |
|
|
31 | Berdnikov (1992e) |
| 2448879.2528 | 0.0154 | |
|
|
83 | Berdnikov, Ibragimov (1994a) |
| 2448879.2664 | 0.0044 | |
|
|
823 | Berdnikov, Ibragimov (1994a) |
| 2449133.4953 | 0.0611 | |
|
|
47 | Arellano Ferro et al. (1998) |
| 2449133.6899 | 0.0450 | |
|
|
59 | Arellano Ferro et al. (1998) |
| 2449221.3067 | 0.0283 | |
|
|
50 | Berdnikov, Ibragimov (1994b) |
| 2449221.3502 | 0.0078 | |
|
|
98 | Berdnikov, Ibragimov (1994b) |
| 2449545.7154 | 0.0098 | |
|
|
164 | Berdnikov et al. (1995) |
| 2449545.7869 | 0.0135 | |
|
|
83 | Berdnikov et al. (1995) |
| 2449817.4896 | 0.0214 | |
|
|
15 | Berdnikov, Turner (1995b) |
| 2449949.1196 | 0.0066 | |
|
|
56 | Berdnikov et al. (1997) |
| 2449962.2720 | 0.0384 | |
|
|
23 | Berdnikov et al. (1997) |
| 2450321.8331 | 0.0152 | |
|
|
133 | Berdnikov et al. (1998) |
| 2450321.8959 | 0.0296 | |
|
|
33 | Berdnikov et al. (1998) |
| 2450370.0671 | 0.0217 | |
|
|
28 | Berdnikov, Turner (1998a) |
| 2450370.1146 | 0.0216 | |
|
|
28 | Berdnikov, Turner (1998a) |
| 2450576.2106 | 0.0143 | |
|
|
35 | Berdnikov, Turner (1998b) |
| 2450812.9499 | 0.0347 | |
|
|
45 | Ignatova, Vozyakova (2000) |
| 2450813.0184 | 0.0300 | |
|
|
45 | Ignatova, Vozyakova (2000) |
| 2450905.0017 | 0.0156 | |
|
|
94 | Berdnikov, Turner (2000) |
| 2450905.2123 | 0.0108 | |
|
|
48 | Berdnikov, Turner (2000) |
| 2451273.2650 | 0.0364 | |
|
|
23 | Berdnikov, Turner (2001) |
| 2451655.0424 | 0.0299 | |
|
|
21 | Berdnikov, Caldwell (2001) |
| 2451655.0956 | 0.0200 | |
|
|
42 | Berdnikov, Caldwell (2001) |
| 2452128.4385 | 0.0219 | |
|
|
71 | ASAS |
| 2452374.1251 | 0.0498 | |
|
|
18 | Berdnikov et al. (2019) |
| 2452584.3868 | 0.0179 | |
|
|
83 | ASAS |
| 2452702.9445 | 0.0220 | |
|
|
66 | Berdnikov et al. (2019) |
| 2453027.2664 | 0.0272 | |
|
|
61 | ASAS |
| 2457175.3384 | 0.0032 | |
|
|
110 | ASAS-SN |
| 2457543.6916 | 0.0046 | |
|
|
99 | ASAS-SN |
| 2457578.8474 | 0.0096 | |
|
|
60 | ASAS-SN |
| 2457798.0453 | 0.0108 | |
|
|
60 | ASAS-SN |
| 2457929.5932 | 0.0028 | |
|
|
154 | ASAS-SN |
| 2458012.8846 | 0.0125 | |
|
|
60 | ASAS-SN |
| 2458253.9979 | 0.0137 | |
|
|
62 | ASAS-SN |
| 2458306.6982 | 0.0041 | |
|
|
120 | ASAS-SN |
| 2458398.7412 | 0.0078 | |
|
|
59 | ASAS-SN |
| 2458438.2560 | 0.0122 | |
|
|
100 | ASAS-SN |
| 2458547.9484 | 0.0091 | |
|
|
80 | ASAS-SN |
| 2458622.4468 | 0.0046 | |
|
|
70 | ASAS-SN |
| 2458675.0362 | 0.0125 | |
|
|
69 | ASAS-SN |
| 2458692.5485 | 0.0108 | |
|
|
60 | ASAS-SN |
| 2458710.1785 | 0.0148 | |
|
|
79 | ASAS-SN |
| 2458727.6876 | 0.0108 | |
|
|
100 | ASAS-SN |
| 2458767.1457 | 0.0044 | |
|
|
70 | ASAS-SN |
| 2458859.1765 | 0.0114 | |
|
|
58 | ASAS-SN |
| 2459017.0831 | 0.0064 | |
|
|
70 | ASAS-SN |
| 2459060.9275 | 0.0125 | |
|
|
71 | ASAS-SN |
| 2459205.5950 | 0.0121 | |
|
|
58 | ASAS-SN |
| 2459227.5498 | 0.0039 | |
|
|
137 | ASAS-SN |
| 2459240.7298 | 0.0105 | |
|
|
79 | ASAS-SN |
| 2459328.3894 | 0.0152 | |
|
|
100 | ASAS-SN |
| 2459359.1290 | 0.0127 | |
|
|
73 | ASAS-SN |
| 2459433.5973 | 0.0109 | |
|
|
57 | ASAS-SN |
| 2459442.4081 | 0.0157 | |
|
|
93 | Sternberg Astronomical Institute |
| 2459442.4219 | 0.0060 | |
|
|
91 | Sternberg Astronomical Institute |
| 2459442.4360 | 0.0231 | |
|
|
95 | Sternberg Astronomical Institute |
| 2459464.3326 | 0.0138 | |
|
|
100 | ASAS-SN |
| 2459530.1323 | 0.0088 | |
|
|
80 | ASAS-SN |
| 2459573.9479 | 0.0065 | |
|
|
70 | ASAS-SN |
| 2459587.0331 | 0.0118 | |
|
|
54 | ASAS-SN |
| 2459731.8671 | 0.0116 | |
|
|
143 | ASAS-SN |
| 2459810.6978 | 0.0183 | |
|
|
52 | ASAS-SN |
| 2459810.7060 | 0.0074 | |
|
|
71 | ASAS-SN |
| 2459823.9061 | 0.0124 | |
|
|
88 | ASAS-SN |
| 2459845.8942 | 0.0164 | |
|
|
78 | ASAS-SN |
Table 5. Quadratic ephemerides in the form
![]() for the
fundamental mode and first overtone of V367 Sct and period change
rates
for the
fundamental mode and first overtone of V367 Sct and period change
rates ![]()
| Oscillation mode | HJD |
|
|
|
| Error | Error | Error | Error | |
| Fundamental tone | 2442301.4091 | 6.293177564 |
|
0.2334 |
| |
|
|
|
|
| First overtone | 2442157.4263 | 4.384747197 |
|
0.2445 |
| |
|
|
|
Table 6. Differences ![]() between the
times of maximum light in the
between the
times of maximum light in the ![]() - and
- and ![]() -band filters relative
to the
-band filters relative
to the ![]() -band filter for the fundamental mode and first
overtone of V367 Sct
-band filter for the fundamental mode and first
overtone of V367 Sct
| Oscillation mode | |
|
| Error | Error | |
| Fundamental tone | |
|
| |
|
|
| First overtone | |
|
| |
|