|
Peremennye Zvezdy (Variable Stars) 45, No. 2, 2025 Received 3 January; accepted 17 January. |
Article in PDF |
|
DOI: 10.24412/2221-0474-2025-45-7-31
|
Mid-infrared Period-luminosity Relations of RR Lyrae Variables via Color-transformed Data
A. K. Dambis, L. N. Berdnikov
Sternberg Astronomical Institute, Moscow State University, Universitetskij pr. 13, Moscow 119992, Russia; leonid.berdnikov@gmail.com
|
We derive RR Lyrae period-metallicity-mid-infrared
luminosity relations in the WISE |
1. Introduction
RR Lyrae variables are old low-mass pulsating core-helium-burning
stars occupying the instability strip. Most of them are
fundamental-mode pulsators (RRab), a smaller fraction pulsates in
the first overtone (RRc), and very few of them pulsate in both
modes simultaneously (RRd). RR Lyraes serve as valuable distance
indicators and kinematical tracers because they obey a rather
tight photometric-band-dependent period-metallicity-luminosity
relations of the form:
There have been many empirical determinations of the parameters of
RR Lyrae period-metallicity-luminosity relations in the
near-infrared bands (![]() ) using both the Baade-Wesselink
method (for relatively bright field stars) and globular-cluster
variables (Fernley et al. 1987; Liu & Janes 1990; Jones et al.
1992; Frolov & Samus 1999; Sollima et al. 2006; Prudil et al.
2024). The problem with the practical application of the near-IR
period-metallicity-luminosity relations for actual population
studies is that the three largest-area near-infrared surveys -
DENIS (Epchtein et al. 1999), 2MASS (Skrutsie et al. 2006), and
VISTA Hemisphere Survey, or VHS (McMahon et al. 2013)
- provide only single-epoch data for this wavelength range. The
multi-epoch near-infrared survey with the most extensive sky
coverage is VVV (Minniti et al. 2024), but it focusses on the
Galactic bulge and a part of the southern Milky-Way disk and thus
covers only 500-plus square degrees.
) using both the Baade-Wesselink
method (for relatively bright field stars) and globular-cluster
variables (Fernley et al. 1987; Liu & Janes 1990; Jones et al.
1992; Frolov & Samus 1999; Sollima et al. 2006; Prudil et al.
2024). The problem with the practical application of the near-IR
period-metallicity-luminosity relations for actual population
studies is that the three largest-area near-infrared surveys -
DENIS (Epchtein et al. 1999), 2MASS (Skrutsie et al. 2006), and
VISTA Hemisphere Survey, or VHS (McMahon et al. 2013)
- provide only single-epoch data for this wavelength range. The
multi-epoch near-infrared survey with the most extensive sky
coverage is VVV (Minniti et al. 2024), but it focusses on the
Galactic bulge and a part of the southern Milky-Way disk and thus
covers only 500-plus square degrees.
The situation has improved substantially in the mid-infrared range
with the advent of the Spitzer (Fazio et al. 2004) and WISE
(Wright et al. 2010) photometric surveys where the latter provides
all-sky multi-epoch coverage and therefore should be best suited
for extensive RR-Lyrae-based Galactic structure and kinematics
studies. However, WISE has two important limitations. First, the
angular resolution, which is equal to 6
![]() 1 and 6
1 and 6
![]() 4 in the
WISE
4 in the
WISE ![]() and
and ![]() bands, respectively (Wright et al. 2010), and
restricts the use of the survey data in crowded fields such as
those of most of globular clusters. Second, the limited
photometric sensitivity, which at the 5
bands, respectively (Wright et al. 2010), and
restricts the use of the survey data in crowded fields such as
those of most of globular clusters. Second, the limited
photometric sensitivity, which at the 5![]() level is
equivalent to 16.6 and 15.6 Vega magnitudes for the
level is
equivalent to 16.6 and 15.6 Vega magnitudes for the ![]() and
and ![]() bands, respectively, with the light curves becoming quite noisy
and scattered already appreciably above these limits: this factor
prevents bona fide study of RR Lyrae stars in all but few of
relatively nearby globular clusters. Such a study was carried out
by Dambis et al. (2014), who calibrated the
period-luminosity-metallicity relations in the WISE
bands, respectively, with the light curves becoming quite noisy
and scattered already appreciably above these limits: this factor
prevents bona fide study of RR Lyrae stars in all but few of
relatively nearby globular clusters. Such a study was carried out
by Dambis et al. (2014), who calibrated the
period-luminosity-metallicity relations in the WISE ![]() and
and
![]() bands based on WISE light curves for 360 and 275 RR Lyraes in
15 and 9 Galactic globular clusters, respectively. Spitzer survey
performs better in both aspects, but it has limited sky coverage
and its data have so far been used to study the period-luminosity
relation in only two globular clusters: Reticulum, which is
located close to the LMC (Muraveva et al. 2018), and the nearby
cluster M4 (Neeley et al. 2015). Mullen et al. (2023) performed
the most comprehensive calibration of RR Lyrae
period-luminosity-metallicity and period-Wesenheit-metallicity
relations based on WISE photometry and Gaia EDR3 parallaxes (Gaia
Collaboration, 2021) of about 1000 mostly relatively bright field
RR Lyraes, thus circumventing the two WISE survey limitations
mentioned above. However, because their study relies of field-star
parallaxes, the accuracy of the inferred period slope estimates is
not as good as those obtained by Dambis et al. (2014) using WISE
data for RR Lyraes in nearby globular clusters despite the rather
large scatter of the period-magnitude relations in individual
clusters due to crowding. Here we propose another way to
circumvent the above two WISE-survey limitations using
intensity-mean WISE
bands based on WISE light curves for 360 and 275 RR Lyraes in
15 and 9 Galactic globular clusters, respectively. Spitzer survey
performs better in both aspects, but it has limited sky coverage
and its data have so far been used to study the period-luminosity
relation in only two globular clusters: Reticulum, which is
located close to the LMC (Muraveva et al. 2018), and the nearby
cluster M4 (Neeley et al. 2015). Mullen et al. (2023) performed
the most comprehensive calibration of RR Lyrae
period-luminosity-metallicity and period-Wesenheit-metallicity
relations based on WISE photometry and Gaia EDR3 parallaxes (Gaia
Collaboration, 2021) of about 1000 mostly relatively bright field
RR Lyraes, thus circumventing the two WISE survey limitations
mentioned above. However, because their study relies of field-star
parallaxes, the accuracy of the inferred period slope estimates is
not as good as those obtained by Dambis et al. (2014) using WISE
data for RR Lyraes in nearby globular clusters despite the rather
large scatter of the period-magnitude relations in individual
clusters due to crowding. Here we propose another way to
circumvent the above two WISE-survey limitations using
intensity-mean WISE ![]() and
and ![]() magnitude estimates computed by
transforming the intensity-mean Gaia
magnitude estimates computed by
transforming the intensity-mean Gaia ![]() and
and ![]() magnitudes. This solution takes advantage of better resolution and
deeper limiting magnitude of the Gaia survey compared to WISE and
therefore makes it possible to study more distant globular
clusters and RR Lyraes in more crowded cluster fields (e.g., stars
located closer to cluster centers).
magnitudes. This solution takes advantage of better resolution and
deeper limiting magnitude of the Gaia survey compared to WISE and
therefore makes it possible to study more distant globular
clusters and RR Lyraes in more crowded cluster fields (e.g., stars
located closer to cluster centers).
2. Calibration formulas
Formula (1) is linear in ![]() and [Fe/H], and hence all
sorts of intensity-mean absolute-magnitude differences, which are
distant independent and equal to the corresponding intensity-mean
color indices (like, e.g.,
and [Fe/H], and hence all
sorts of intensity-mean absolute-magnitude differences, which are
distant independent and equal to the corresponding intensity-mean
color indices (like, e.g.,
![]() , (
, (
![]() , etc.), and linear combinations thereof also obey
similar linear relations of the same form. As a consequence, the
same is true for extinction-free
, etc.), and linear combinations thereof also obey
similar linear relations of the same form. As a consequence, the
same is true for extinction-free ![]() indices constructed from
observed colors (with coefficients chosen so as to cancel out
extinction). With the Cardelli et al. (1989) and O'Donnell (1994)
extinction law and assuming
indices constructed from
observed colors (with coefficients chosen so as to cancel out
extinction). With the Cardelli et al. (1989) and O'Donnell (1994)
extinction law and assuming ![]() =3.1, the extinction ratios are
equal to:
=3.1, the extinction ratios are
equal to:
and
and
and
3. Calibration sample
Our calibrating sample is based on two RR Lyrae star
lists with spectroscopic metallicity estimates. One is the subset
of the catalog of Liu et al. (2020) including 5206 RR Lyraes with
both metallicity and metallicity error estimates derived from
spectra acquired within the framework of the LAMOST (Deng et al.
2012; Liu et al. 2014) and SDSS (SEGUE) (Yanni et al. 2009)
surveys and assumed to be in the UVES
high-resolution-spectroscopy-based scale (Carretta et al. 2009).
The second list is the catalog of 850 RR Lyraes by Muhie et al.
(2021) with metallicities based on (1) original [Fe/H] estimates
determined from low-resolution spectra taken with the 11-m SALT
(Southern African Large Telescope) (Buckley 2006; O'Donoghue et
al. 2006) in the process of the Milky wAy Galaxy wIth SALT
spectroscopy (MAGIC) project (Kniazev et al. 2019) and (2)
published spectroscopic [Fe/H] determinations compiled and
homogenized by Dambis et al. (2013). The metallicities in the
catalog of Muhie et al. (2021) are in the old Zinn and West (1984)
(ZW) scale. We use the formula from Carretta et al. (2009):
to transform them to the UVES scale adopted by Liu et al. (2020). The two lists have 139 stars in common, for which we adopt the [Fe/H] values from the more homogeneous catalog of Liu et al. (2020), with the combined list containing 5717 stars. We then cross-matched this combined list with the Clementini et al. (2023) calalog of stars classified as RR Lyrae variables based on an analysis of epoch photometry provided in Gaia DR3 (Gaia Collaboration 2023) and with ALLWISE Multiepoch Photometry and NEOWISE-R Single Exposure (L1b) Source Tables to extract the
and
The corresponding fits for 335 first-overtone RR Lyraes (RRc) are:
and
Equations (7) - (13) imply the following transformation formulas:
and
for RRab- and RRd-type variables and:
and
for RRc-type variables. We point out two important properties of the above formulas: (1) their rather small scatter (about 0
4. Calibration of the Period-Metallicity-Luminosity relation
4.1. The period slope
We start by determining the period slopes (derived by fitting homogenized intensity-mean
To estimate the period slope ![]() or
or ![]() for a
particular cluster, we use the procedure employed by Dambis et al.
(2014) and just slightly modify the final step. The revised
procedure goes as follows. Given that stars in a cluster are
located practically at the same distance and (generally) have the
same metallicity and the same amount of interstellar extinction
(in any case, the latter is at least 17 times smaller in all WISE
passbands compared to the
for a
particular cluster, we use the procedure employed by Dambis et al.
(2014) and just slightly modify the final step. The revised
procedure goes as follows. Given that stars in a cluster are
located practically at the same distance and (generally) have the
same metallicity and the same amount of interstellar extinction
(in any case, the latter is at least 17 times smaller in all WISE
passbands compared to the ![]() band and therefore its variations
can therefore be ignored), equation (1) becomes:
band and therefore its variations
can therefore be ignored), equation (1) becomes:
which is just a rewritten form of equation (18), for stars with
Here, like in Dambis et al. (2014), we plot the scaled computed
intensity-mean ![]() and
and ![]() magnitudes (
magnitudes (![]() and
and
![]() ) for our calibrating clusters as a function of
fundamentalized periods (Figs. (3-6). As it is evident from
Table 1 and Figs. (3-6), the period-magnitude relation slopes
are quite consistent among the clusters studied. Moreover, one can
see from Figs. (7) and (8) that the slopes show only a marginal
trend with metallicity: the corresponding linear weighted fits
are:
) for our calibrating clusters as a function of
fundamentalized periods (Figs. (3-6). As it is evident from
Table 1 and Figs. (3-6), the period-magnitude relation slopes
are quite consistent among the clusters studied. Moreover, one can
see from Figs. (7) and (8) that the slopes show only a marginal
trend with metallicity: the corresponding linear weighted fits
are:
The trends differ from zero by 1.4 ![]() , and, interestingly,
they are in the sense opposite to that found by Dambis et
al. (2014). We hereafter compute the combined solutions assuming
the same slope for all clusters and using weights based on the
scatter of individual-cluster PL solutions listed in Table 1.
These combined solutions yield the slopes of
, and, interestingly,
they are in the sense opposite to that found by Dambis et
al. (2014). We hereafter compute the combined solutions assuming
the same slope for all clusters and using weights based on the
scatter of individual-cluster PL solutions listed in Table 1.
These combined solutions yield the slopes of
![]() and
and
![]() for the
for the ![]() - and
- and ![]() -band relations,
respectively. Figs. (9) and (10) show the combined
-band relations,
respectively. Figs. (9) and (10) show the combined ![]() versus
versus
![]() and
and ![]() versus
versus
![]() plots for RR Lyraes passing the above filters in the 24
globular clusters of the final list. We list the
plots for RR Lyraes passing the above filters in the 24
globular clusters of the final list. We list the ![]() and
and
![]() parameters obtained from these combined solutions in the
last two columns of Table 1.
parameters obtained from these combined solutions in the
last two columns of Table 1.
4.2. Intrinsic color calibration
To finalize our calibration of the mid-infrared period-metallicity-luminosity relations for RR Lyrae stars and to use it to compute distances to these variables, we need to be able to estimate interstellar extinction to individual stars. To this end, we now derive a calibration for theHence
or (in view of
and
We use formula (22) and
Given the extinction ratios (2), the total extinction in the
and
respectively. The scatter of intrinsic color
4.3. The metallicity slope and zero point
To estimate the two remaining parameters of the
period-metallicity-luminosity relation (1), ![]() and
and
![]() , we cross-match our initial calibration sample of 5717
RR Lyraes having spectroscopic metallicity estimates with the Gaia
DR3-based catalog of RR Lyrae-type variables by Clementini et al.
(2023) and leave only stars for which the latter catalog provides
both
, we cross-match our initial calibration sample of 5717
RR Lyraes having spectroscopic metallicity estimates with the Gaia
DR3-based catalog of RR Lyrae-type variables by Clementini et al.
(2023) and leave only stars for which the latter catalog provides
both ![]() - and
- and ![]() -band intensity-mean magnitudes. We use
formulas (14)-(17) and (23)-(25) to compute the dereddened
-band intensity-mean magnitudes. We use
formulas (14)-(17) and (23)-(25) to compute the dereddened ![]() -
and
-
and ![]() -band intensity-mean magnitudes of the stars considered.
We make further astrometric and photometric quality cuts leaving
only stars with RUWE
-band intensity-mean magnitudes of the stars considered.
We make further astrometric and photometric quality cuts leaving
only stars with RUWE ![]() , astrometric_excess_noise
, astrometric_excess_noise ![]() , corrected
, corrected ![]() and
and ![]() flux excess factor
flux excess factor ![]() . A
total of 4658 stars pass these cuts.
. A
total of 4658 stars pass these cuts.
Like Mullen et al. (2024), we determine ![]() and
and ![]() by
fitting period-metallicity-luminosity relation using the
Astrometric Based Luminosity (ABL)
by
fitting period-metallicity-luminosity relation using the
Astrometric Based Luminosity (ABL) ![]() :
:
respectively. Ordinary 4
and
The standard unit-weight error is equal to 1.20 in both cases, indicating that the quoted Gaia DR3 parallax errors appear to be slightly underestimated.
However, in our case there are also significant errors in
independent variables [Fe/H], and simple regression fits produce
biased results. To estimate and correct the biases introduced, we
generate two sets of 400 simulated 4658-star samples with the
parallax
![]() of each star computed using
formulas (27) and (28) based on observed
of each star computed using
formulas (27) and (28) based on observed ![]() , [Fe/H], and
, [Fe/H], and
![]() or
or ![]() values and with
values and with ![]() ,
, ![]() ,
,
![]() , and
, and ![]() parameters inferred above, adding
simulated random noise normally distributed with zero mean and
errors computed based on quoted Gaia DR3 parallax errors and
0
parameters inferred above, adding
simulated random noise normally distributed with zero mean and
errors computed based on quoted Gaia DR3 parallax errors and
0![]() 05 scatter in
05 scatter in ![]() or
or ![]() magnitudes and then multiplied
by 1.2. The simulated metrallicities [Fe/H] are equal to the
observed ones plus random noise normally distributed with zero
mean and quoted metallicity errors. We found the bias
magnitudes and then multiplied
by 1.2. The simulated metrallicities [Fe/H] are equal to the
observed ones plus random noise normally distributed with zero
mean and quoted metallicity errors. We found the bias ![]() and
and ![]() in the sense the estimated minus true
value to be
in the sense the estimated minus true
value to be
and
implying the following bias-corrected values:
and
5. Results and validation
Thus, our finaland
with standard errors about 0
and
and differ by much higher accuracy of the period slopes and slightly steeper metallicity slopes. Note that our
(we recentered it to
We use relations (31) and (32) combined with the
![]() intrinsic-color calibration (23) and
extinction ratios (2) to compute the true distance moduli
(
intrinsic-color calibration (23) and
extinction ratios (2) to compute the true distance moduli
(![]() (W1) and
(W1) and ![]() (W2)), the corresponding photometric
parallaxes (
(W2)), the corresponding photometric
parallaxes (![]() and
and ![]() ), and distances
(
), and distances
(![]() and
and ![]() ) for the 24 globular clusters of our final
list, and compare them to the globular-cluster trigonometric
parallaxes determined via Gaia EDR3 parallaxes and kinematic
distances found from Gaia EDR3 or Hubble Space Telescope
proper-motion dispersion profiles combined with radial-velocity
dispersion profiles (Baumgardt & Vasiliev 2021). We summarize all
these data in Table 2. Given that the errors of our
globular-cluster photometric parallaxes and photometric distances
are mush smaller than those of the cluster trigonometric
parallaxes and kinematic distances reported by Baumgardt &
Vasiliev (2021), we treat the former in the following weighted
regression fits as independent variables with no errors and the
latter as dependent variables with the corresponding quoted
errors:
) for the 24 globular clusters of our final
list, and compare them to the globular-cluster trigonometric
parallaxes determined via Gaia EDR3 parallaxes and kinematic
distances found from Gaia EDR3 or Hubble Space Telescope
proper-motion dispersion profiles combined with radial-velocity
dispersion profiles (Baumgardt & Vasiliev 2021). We summarize all
these data in Table 2. Given that the errors of our
globular-cluster photometric parallaxes and photometric distances
are mush smaller than those of the cluster trigonometric
parallaxes and kinematic distances reported by Baumgardt &
Vasiliev (2021), we treat the former in the following weighted
regression fits as independent variables with no errors and the
latter as dependent variables with the corresponding quoted
errors:
Note that an analysis involving eventual bias in globular-cluster
trigononetric parallax estimates reported by Baumgardt & Vasiliev
(2021) yields:
(24 clusters), i.e. our photometric parallaxes of globular clusters appear to be totally consistent with estimates based on trigonometric parallaxes of individual cluster stars, and the unaccounted bias in globular-cluster parallaxes reported by Baumgardt & Vasiliev (2021) appears to be of about
We finally test our approach by applying to it to faint Gaia
RR Lyrae variables. To this end, we cross-match the catalog of
Clementini et al. (2023) with the list of Cusano et al. (2021)
containing about 22000 RR Lyraes in the Large Magellanic Cloud
having near-infrared photometry light curves from VISTA survey of
the Magellanic Clouds system (VMC) and optical data from the
Optical Gravitational Lensing Experiment (OGLE) IV survey and the
Gaia Data Release 2 catalogue, which the authors consider to be
LMC members. We leave only stars with corrected ![]() and
and ![]() flux excess factor
flux excess factor ![]() with our final list containing a
total of 11901 stars for which we compute the WISE
with our final list containing a
total of 11901 stars for which we compute the WISE ![]() - and
- and
![]() -band intensity-mean magnitudes
-band intensity-mean magnitudes ![]() and
and ![]() via
formulas (14)-(17) above adopting me same [Fe/H]
via
formulas (14)-(17) above adopting me same [Fe/H]![]() equal to
the median value for LMC fundamental-mode RR Lyraes (Skowron et
al. 2016). We do not use individual-star metallicity estimates
from Cusano et al. (2021) because they are unavailable for a large
fraction of the list and those available have errors comparable to
or greater than the scatter of the values themselves. We then
deredden
equal to
the median value for LMC fundamental-mode RR Lyraes (Skowron et
al. 2016). We do not use individual-star metallicity estimates
from Cusano et al. (2021) because they are unavailable for a large
fraction of the list and those available have errors comparable to
or greater than the scatter of the values themselves. We then
deredden ![]() and
and ![]() by substracting
by substracting ![]() =0.487
=0.487![]() and
and ![]() =0.294
=0.294![]() extinction values, respectively, where
extinction values, respectively, where
![]() -band extinction estimates
-band extinction estimates ![]() are adopted from Cusano et al.
(2021) and the extinction ratios are computed based on the
Cardelli et al. (1989) and O'Donnell (1994) extinction law and
assuming
are adopted from Cusano et al.
(2021) and the extinction ratios are computed based on the
Cardelli et al. (1989) and O'Donnell (1994) extinction law and
assuming ![]() =3.1. The 3-
=3.1. The 3-![]() clipped regression fits of the
period-magnitude relations
clipped regression fits of the
period-magnitude relations
![]() and
and
![]() are:
are:
Note that, despite the large scatter (which is to be expected for stars at the faint end of the Gaia catalog and located in a very crowded field), the slopes are remarkably close to the values inferred above from much brighter globular-cluster stars. Our relations (31) and (32), combined with the
6. Conclusions
We derived color transformations permitting the intensity-mean WISE
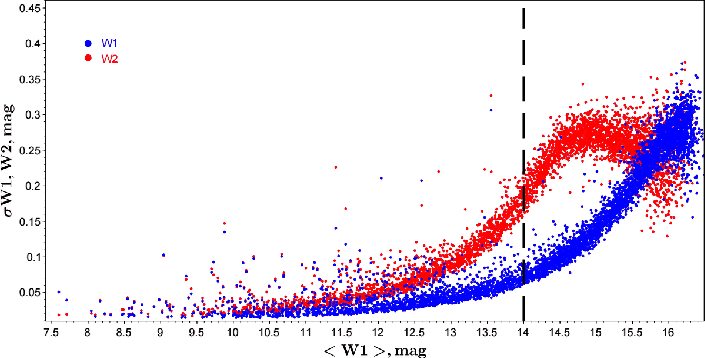 |
Fig. 1.
The rms scatter of
the W1 (blue fots) and W2 (red dots) data points about the light
curve as a function of |
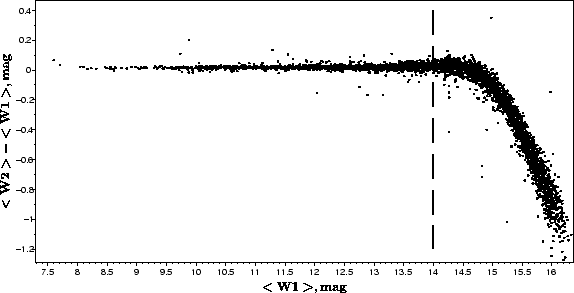 |
Fig. 2.
The |
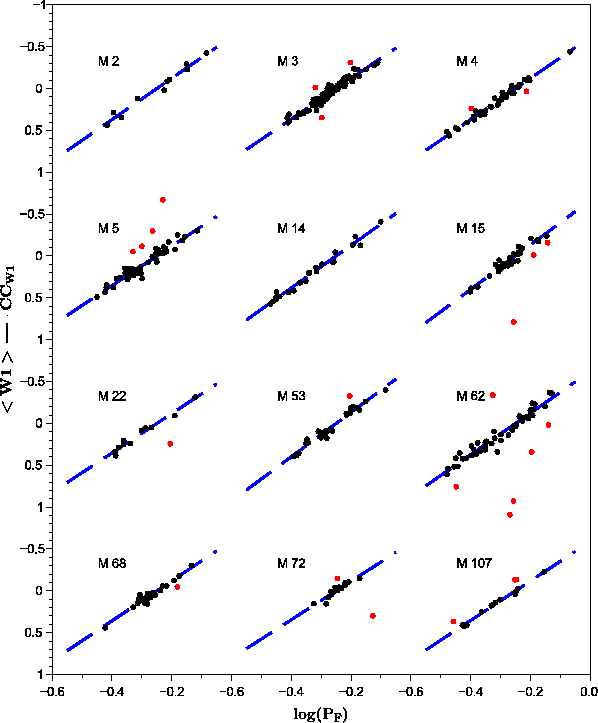 |
Fig. 3.
The
PL-(transformed |
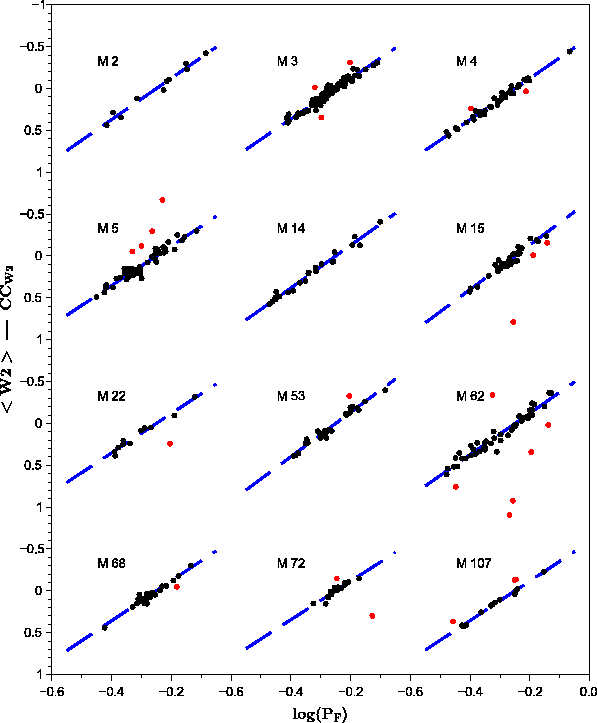 |
Fig. 5.
The
PL-(transformed |
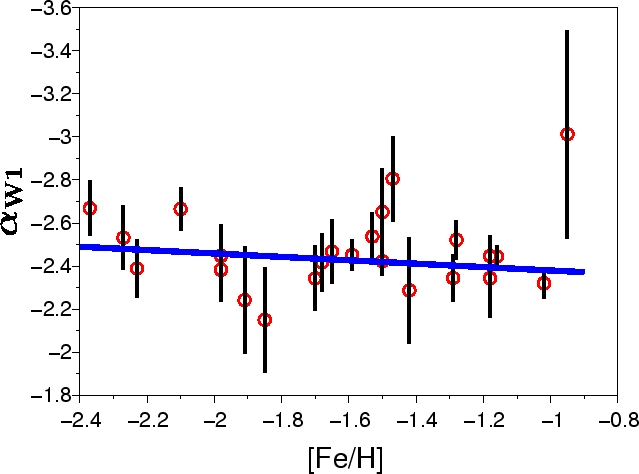 |
Fig. 7.
The period slope
|
 |
Fig. 8.
The period slope
|
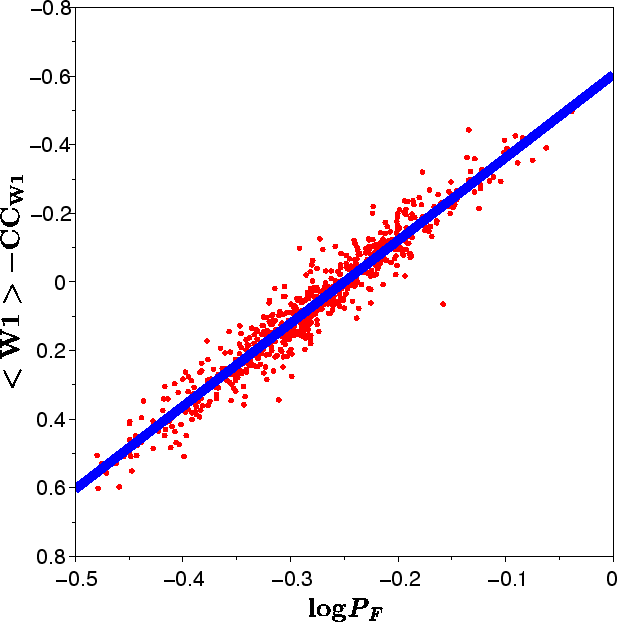 |
Fig. 9.
The combined
period- |
 |
Fig. 10.
The combined
period- |
7. Acknowledgments
The study was conducted under the state assignment of Lomonosov Moscow State University.This work has made use of data from the European Space Agency (ESA) mission Gaia (https://www.cosmos.esa.int/gaia), processed by the Gaia Data Processing and Analysis Consortium (DPAC, https://www.cosmos.esa.int/web/gaia/dpac/consortium). Funding for the DPAC has been provided by national institutions, in particular the institutions participating in the Gaia Multilateral Agreement.
This publication makes use of data products from the Wide-field Infrared Survey Explorer, which is a joint project of the University of California, Los Angeles, and the Jet Propulsion Laboratory/California Institute of Technology, funded by the National Aeronautics and Space Administration.
This publication also makes use of data products from NEOWISE, which is a project of the Jet Propulsion Laboratory/California Institute of Technology, funded by the Planetary Science Division of the National Aeronautics and Space Administration.
References:
Baumgardt, H. & Vasiliev, E. 2021, Monthly Notices Roy. Astron. Soc., 505, No. 4, 5957
Bhardwaj, A., Marconi, M., Rejkuba, M., et al. 2023, Astrophys. J. Lett., 944, No. 2, L51
Bono, G., Caputo, F., Castellani, V., et al. 2003, Monthly Notices Roy. Astron. Soc., 344, No. 4, 1097
Buckley, D. A. H., Swart, G. P., Meiring, J. G., 2006, Proceedings of the SPIE, 6267, 62670Z
Butler, D. J. 2003, Astron. & Astrophys., 405, No. 3, 981
Cardelli. J. A., Clayton, G. C., & Mathis J. S. 1989,Astrophys. J., 345, No. 1, 245
Carretta, E., Bragaglia, A., Gratton, R., et al. 2009, Astron. & Astrophys., 508, No. 2, 695
Cassisi, S., Castellani, M., Caputo, F., & Castellani, V. 2004, Astron. & Astrophys., 426, No. 2, 641
Catelan, M., Pritzl, B. J., & Smith, H. A. 2004, Astrophys. J., Suppl. Ser., 154, No. 2, 633
Clementini, G., Ripepi, V., Garofalo, A., et al. 2023, Astron. & Astrophys., 674, article id. A18
Cusano, F., Moretti, M. I., Clementini, G., et al. 2021, Monthly Notices Roy. Astron. Soc., 504, No. 1, 1
Dambis, A., Berdnikov, L., Kniazev, A. Y., et al. 2013, Monthly Notices Roy. Astron. Soc., 435, No. 4, 3206
Dambis, A. K., Rastorguev, A. S., & Zabolotskikh, M. V. 2014, Monthly Notices Roy. Astron. Soc., 439, No. 4, 3765
Deng, L.-C., Newberg, H. J., Liu, C., et al., 2012, Res. Astron. Astrophys., 12, No. 7, 735
de Jong, J. T. A., Yanny, B., Rix, H.-W., et al. 2010, Astrophys. J., 714, No. 1, 663
Epchtein, N., Deul, E., Derriere, S., et al. 1999, Astron. & Astrophys., 349, No. 1, 236
Evans, D. W., Riello, M., De Angeli, F., et al. 2018, Astron. & Astrophys., 616, article id. A4
Fazio, G. G., Hora, J. L., Allen, L. E., et al. 2004, Astrophys. J., Suppl. Ser., 154, No. 1, 10
Fernley, J. A., Longmore, A. J., & Jameson, R. F. 1987, Lecture Notes in Physics, 274, 239
Frolov, M. S. & Samus, N. N., 1998, Astron. Lett., 24, No. 2. 171
Gaia Collaboration, Prusti, T., de Bruijne, J. H. L., Brown, A. G. A., et al. 2016, Astron. & Astrophys., 595, article id. A1
Gaia Collaboration, Brown, A. G. A., Vallenari, A., Prusti, T., et al. 2021, Astron. & Astrophys., 649, article id. A1
Gaia Collaboration, Brown, A. G. A., Vallenari, A., Prusti, T., et al. 2021, Astron. & Astrophys., 649, article id. A1
Gaia Collaboration, Vallenari, A., Brown, A. G. A., Prusti, T. et al. 2023, Astron. & Astrophys., 674, article id. A1
Harris, W. E. 2010, arXiv:1012.3224
Iben Jr., I. 1974, Ann. Rev. Astron. & Astrophys., 12, 215
Jones, R. V., Carney, B. W., Storm, J., & Latham, D. W. 1992, Astrophys. J., 386, No. 2, 646
Kniazev, A. Y., Usenko, I. A., Kovtyukh, V. V., & Berdnikov, L. N. 2019, Astrophys. Bull., 74, No. 2, 208
Lindegren, L., Bastian, U., Biermann, M., et al. 2021, Astron. & Astrophys., 649, article id. A4
Liu, T. & Janes, K. A., 1990, Astrophys. J., 354, No. 1, 273
Liu, X.-W., Yuan, H.-B., Huo, Z.-Y., et al. 2014, IAUS, 298, 310
Liu, G.-C., Huang, Y., Zhang, H.-W., et al. 2020, Astrophys. J., Suppl. Ser., 247, article id. 68
Marconi, M., Coppola, G., Bono, G., et al. 2015, Astrophys. J., 808, No. 1, article id. 50
McMahon, R. G., Banerji, M., Gonzalez, E., et al. 2013, Messenger, 154, 35
Minniti, D., Lucas, P. W., Emerson, J. P., et al. 2010, New Astron., 15, 433
Muhie T. D., Dambis A. K., Berdnikov L. N., et al. 2021, Monthly Notices Roy. Astron. Soc., 502, No. 3, 4074
Mullen, J. P., Marengo, M., Martínez-Vázquez, C. E., et al. 2023, Astrophys. J., 945, No. 1, article id. 83
Muraveva, T., Garofalo, A., Scowcroft, V., et al. 2018, Monthly Notices Roy. Astron. Soc., 480, No. 3, 4138
Neeley, J. R., Marengo, M., Bono, G., et al. 2015, Astrophys. J., 808, No. 1, article id. 11
Neeley, J. R., Marengo, M., Bono, G. et al. 2017, Astrophys. J., 841, No. 2, article id. 84
O'Donoghue, D., Buckley, D. A. H., Balona, L. A., et al. 2006, Monthly Notices Roy. Astron. Soc., 372, No. 1, 151
O'Donnell, J. E. 1994, Astrophys. J., 422, No. 1, 158
Pietrzynski, G., Graczyk, D., Gallenne, A., et al. 2019, Nature, 567, No. 7747, 200
Riello, M., De Angeli, F., Evans, D. W., et al. 2021, Astron. & Astrophys., 649, article id. A3
Skowron, D. M., Soszynski, I., Udalski, A., et al. 2016, Acta Astron., 66, No. 3, 269
Skrutskie, M. F., Cutri, R. M., Stiening, R., et al. 2006, Astron. J., 131, No. 2, 1163
Sollima, A., Cacciari, C., & Valenti, E. 2006, Monthly Notices Roy. Astron. Soc., 372, No. 3, 1675
Wright, E. L., Eisenhardt, P. R. M., Mainzer, A. K., et al. 2010, Astron. J., 140, No. 6, 1868
Yanny, B., Rockosi, C., Newberg, H. J., et al. 2009, Astron. J., 137, No. 5, 4377
Yuan, H. B., Liu, X. W., & Xiang, M. S. 2013, Monthly Notices Roy. Astron. Soc., 430, No. 3, 2188
Zinn, R. & West, M. J., 1984, Astrophys. J., Suppl. Ser., 55, No. 1, 45
Table 1. Parameters of the
![]() =
=
![]() + 0.25) +
+ 0.25) + ![]() and
and ![]() =
=
![]() + 0.25) +
+ 0.25) + ![]() fits for globular clusters
with at least eight RR Lyraes outlining linear relation
fits for globular clusters
with at least eight RR Lyraes outlining linear relation
| Cluster | Alt. | |
[Fe/H] | |
|
|
|
|
|
|
|
|
| name | name | ( |
( |
|||||||||
| -2.418) | -2.449) | |||||||||||
| NGC 7089 | M 2 | 0.06 | -1.65 | 10 | 14.804 | -2.431 | 0.048 | 14.824 | -2.468 | 0.040 | 14.804 | 14.824 |
| |
|
|
|
|
|
|||||||
| NGC 5272 | M 3 | 0.01 | -1.50 | 93 | 14.485 | -2.386 | 0.037 | 14.506 | -2.422 | 0.038 | 14.484 | 14.505 |
| |
|
|
|
|
|
|||||||
| NGC 6121 | M 4 | 0.35 | -1.16 | 43 | 10.959 | -2.412 | 0.029 | 10.953 | -2.443 | 0.029 | 10.959 | 10.952 |
| |
|
|
|
|
|
|||||||
| NGC 5904 | M 5 | 0.03 | -1.29 | 44 | 13.822 | -2.321 | 0.054 | 13.843 | -2.344 | 0.056 | 13.817 | 13.838 |
| |
|
|
|
|
|
|||||||
| NGC 6402 | M 14 | 0.60 | -1.28 | 22 | 14.343 | -2.502 | 0.041 | 14.325 | -2.521 | 0.043 | 14.349 | 14.331 |
| |
|
|
|
|
|
|||||||
| NGC 7078 | M 15 | 0.10 | -2.37 | 34 | 14.469 | -2.601 | 0.040 | 14.483 | -2.668 | 0.041 | 14.473 | 14.488 |
| |
|
|
|
|
|
|||||||
| NGC 6656 | M 22 | 0.34 | -1.70 | 11 | 12.095 | -2.313 | 0.037 | 12.093 | -2.342 | 0.040 | 12.088 | 12.087 |
| |
|
|
|
|
|
|||||||
| NGC 5024 | M 53 | 0.02 | -2.10 | 30 | 15.766 | -2.604 | 0.039 | 15.787 | -2.665 | 0.041 | 15.768 | 15.789 |
| |
|
|
|
|
|
|||||||
| NGC 6266 | M 62 | 0.47 | -1.18 | 46 | 13.496 | -2.439 | 0.060 | 13.484 | -2.446 | 0.062 | 13.493 | 13.479 |
| |
|
|
|
|
|
|||||||
| NGC 4590 | M 68 | 0.05 | -2.23 | 26 | 14.414 | -2.330 | 0.035 | 14.431 | -2.388 | 0.036 | 14.412 | 14.430 |
| |
|
|
|
|
|
|||||||
| NGC 6981 | M 72 | 0.05 | -1.42 | 15 | 15.601 | -2.267 | 0.031 | 15.621 | -2.286 | 0.033 | 15.601 | 15.620 |
| |
|
|
|
|
|
|||||||
| NGC 6171 | M 107 | 0.33 | -1.02 | 12 | 13.258 | -2.305 | 0.017 | 13.253 | -2.320 | 0.018 | 13.250 | 13.244 |
| |
|
|
|
|
|
|||||||
| NGC 1851 | 0.02 | -1.18 | 10 | 14.855 | -2.325 | 0.050 | 14.876 | -2.343 | 0.052 | 14.848 | 14.867 | |
| |
|
|
|
|
|
|||||||
| NGC 3201 | 0.24 | -1.59 | 67 | 12.834 | -2.405 | 0.028 | 12.838 | -2.451 | 0.030 | 12.834 | 12.837 | |
| |
|
|
|
|
|
|||||||
| NGC 4833 | 0.32 | -1.85 | 9 | 13.492 | -2.127 | 0.053 | 13.492 | -2.149 | 0.054 | 13.500 | 13.500 | |
| |
|
|
|
|
|
|||||||
| NGC 5053 | 0.01 | -2.27 | 9 | 15.567 | -2.476 | 0.040 | 15.588 | -2.532 | 0.041 | 15.566 | 15.587 | |
| |
|
|
|
|
|
|||||||
| NGC 5466 | 0.00 | -1.98 | 19 | 15.391 | -2.339 | 0.054 | 15.412 | -2.382 | 0.055 | 15.392 | 15.413 | |
| |
|
|
|
|
|
|||||||
| NGC 5824 | 0.13 | -1.91 | 24 | 16.991 | -2.200 | 0.101 | 17.004 | -2.241 | 0.104 | 16.984 | 16.997 | |
| |
|
|
|
|
|
|||||||
| NGC 6101 | 0.05 | -1.98 | 13 | 15.162 | -2.396 | 0.042 | 15.177 | -2.447 | 0.043 | 15.161 | 15.177 | |
| |
|
|
|
|
|
|||||||
| NGC 6584 | 0.10 | -1.50 | 25 | 15.089 | -2.617 | 0.081 | 15.104 | -2.651 | 0.082 | 15.097 | 15.112 | |
| |
|
|
|
|
|
|||||||
| NGC 6638 | 0.41 | -0.95 | 9 | 14.385 | -2.983 | 0.092 | 14.377 | -3.011 | 0.093 | 14.450 | 14.442 | |
| |
|
|
|
|
|
|||||||
| NGC 6934 | 0.10 | -1.47 | 17 | 15.426 | -2.782 | 0.050 | 15.431 | -2.805 | 0.069 | 15.425 | 15.431 | |
| |
|
|
|
|
|
|||||||
| IC 4499 | 0.23 | -1.53 | 72 | 15.888 | -2.500 | 0.057 | 15.894 | -2.537 | 0.058 | 15.889 | 15.895 | |
| |
|
|
|
|
|
|||||||
| NGC 5139 | |
0.12 | -1.68 |
33 | 13.140 | -2.371 | 0.061 | 13.158 | -2.416 | 0.062 | 13.140 | 13.153 |
| |
|
|
|
|
|
![]() Because of the metallicity spread
among RR Lyrae stars in this cluster (Sollima et al.
2006 and reference therein), we left only the metal-poor stars
(
Because of the metallicity spread
among RR Lyrae stars in this cluster (Sollima et al.
2006 and reference therein), we left only the metal-poor stars
(
![]() corresponding to
corresponding to
![]() ).
).
Table 2. Comparison of our RR Lyrae-based photometric distance estimates to 24 globular clusters with Gaia DR3-based trogonometric parallax estimates and Gaia DR3- and HST-based kinematical distance estimates from Vasiliev & Baumgardt (2021)
| Cluster | Alt. | |
|
|
|
|
|
|
|
|
| name | name | (W1) | kpc | mas | (W2) | kpc | |
|
kpc | kpc |
| NGC 7089 | M 2 | 15.288 | 11.416 | 87.64 | 15.290 | 11.429 | 87.50 | 82 | 11.940 | |
| |
|
|
|
|
|
|
|
|||
| NGC 5272 | M 3 | 14.951 | 9.778 | 102.27 | 14.951 | 9.778 | 102.27 | 110 | 10.116 | |
| |
|
|
|
|
|
|
|
|||
| NGC 6121 | M 4 | 11.310 | 1.828 | 547.04 | 11.304 | 1.823 | 548.57 | 556 | 1.878 | |
| |
|
|
|
|
|
|
|
|||
| NGC 5904 | M 5 | 14.246 | 7.067 | 141.50 | 14.246 | 7.065 | 141.53 | 141 | 7.467 | 7.456 |
| |
|
|
|
|
|
|
|
|
||
| NGC 6402 | M 14 | 14.676 | 8.616 | 116.06 | 14.677 | 8.617 | 116.06 | 129 | ||
| |
|
|
|
|
|
|
||||
| NGC 7078 | M 15 | 15.069 | 10.322 | 96.88 | 15.072 | 10.337 | 96.73 | 97 | 10.375 | |
| |
|
|
|
|
|
|
|
|||
| NGC 6656 | M 22 | 12.531 | 3.207 | 311.78 | 12.532 | 3.209 | 311.65 | 306 | 3.181 | 3.161 |
| |
|
|
|
|
|
|
|
|
||
| NGC 5024 | M 53 | 16.333 | 18.475 | 54.13 | 16.336 | 18.504 | 54.04 | 67 | 17.313 | |
| |
|
|
|
|
|
|
|
|||
| NGC 6266 | M 62 | 13.826 | 5.824 | 171.70 | 13.822 | 5.812 | 172.04 | 185 | 6.395 | 6.502 |
| |
|
|
|
|
|
|
|
|
||
| NGC 4590 | M 68 | 14.994 | 9.970 | 100.30 | 14.995 | 9.979 | 100.02 | 113 | ||
| |
|
|
|
|
|
|
||||
| NGC 6981 | M 72 | 16.048 | 16.203 | 61.72 | 16.048 | 16.207 | 61.70 | 84 | ||
| |
|
|
|
|
|
|
||||
| NGC 6171 | M 107 | 13.581 | 5.203 | 192.20 | 13.574 | 5.187 | 192.79 | 194 | 6.017 | |
| |
|
|
|
|
|
|
|
|||
| NGC 1851 | 15.260 | 11.273 | 88.70 | 15.258 | 11.260 | 88.81 | 88 | |||
| |
|
|
|
|
|
|
||||
| NGC 3201 | 13.276 | 4.520 | 221.24 | 13.274 | 4.517 | 221.37 | 222 | 4.745 | ||
| |
|
|
|
|
|
|
|
|||
| NGC 4833 | 13.971 | 6.226 | 160.62 | 13.972 | 6.230 | 160.52 | 164 | 5.822 | ||
| |
|
|
|
|
|
|
|
|||
| NGC 5053 | 16.161 | 17.071 | 58.58 | 16.163 | 17.088 | 58.52 | 50 | |||
| |
|
|
|
|
|
|
||||
| NGC 5466 | 15.941 | 15.427 | 64.82 | 15.942 | 15.430 | 64.81 | 57 | |||
| |
|
|
|
|
|
|
||||
| NGC 5824 | 17.499 | 31.604 | 31.64 | 17.500 | 31.629 | 31.62 | 57 | |||
| |
|
|
|
|
|
|
||||
| NGC 6101 | 15.702 | 13.814 | 72.39 | 15.700 | 13.805 | 72.44 | 84 | |||
| |
|
|
|
|
|
|
||||
| NGC 6584 | 15.548 | 12.872 | 77.68 | 15.548 | 12.872 | 77.69 | 77 | |||
| |
|
|
|
|
|
|
||||
| NGC 6638 | 14.756 | 8.936 | 111.90 | 14.752 | 8.920 | 112.10 | 115 | |||
| |
|
|
|
|
|
|
||||
| NGC 6934 | 15.872 | 14.944 | 66.92 | 15.862 | 14.872 | 67.24 | 78 | 16.718 | ||
| |
|
|
|
|
|
|
|
|||
| IC 4499 | 16.323 | 18.388 | 54.38 | 16.322 | 18.387 | 54.39 | 54 | |||
| |
|
|
|
|
|
|
||||
| NGC 5139 | |
13.617 | 5.291 | 188.99 | 13.618 | 5.293 | 188.93 | 193 | 5.359 | 5.264 |
| |
|
|
|
|
|
|
|
|

