|
Peremennye Zvezdy (Variable Stars) 45, No. 5, 2025 Received 3 January; accepted 17 February. |
Article in PDF |
|
DOI: 10.24412/2221-0474-2025-45-54-62
|
Results of Light Curves Analysis for Eclipsing Old Nova V728 Scorpii in Active and Quiet States
I. B. Voloshina1, T. S. Khruzina1, A. N. Tarasenkov1,2
- Sternberg Astronomical Institute, Moscow State University,
Universitetskij pr. 13, Moscow 119992, Russia
- Institute of Astronomy of Russian Academy of Sciences, 48 Pyatnitskaya Str., Moscow 119017, Russia
|
The results of light curve analysis are presented for
the old Nova V728 Sco. The object is a high-inclination system
with an orbital period of
|
1. History of the variable
The cataclysmic variable V728 Sco (N Sco 1862, also known as N Ara
1862) was discovered on October 4, 1862 by Tebbutt (1878) at the
brightness of
![]() . Later it was identified with an
eclipsing dwarf nova with coordinates
. Later it was identified with an
eclipsing dwarf nova with coordinates
![]() (J2000;
Tappert et al. 2012). The Gaia-DR3 coordinates of this star are
very similar:
(J2000;
Tappert et al. 2012). The Gaia-DR3 coordinates of this star are
very similar:
![]() , equinox and epoch J2000.0. The
outbursts in this system occur every
, equinox and epoch J2000.0. The
outbursts in this system occur every
![]() with mean
duration about 10
with mean
duration about 10![]() . The brightness of V728 Sco during dwarf
nova outbursts was
. The brightness of V728 Sco during dwarf
nova outbursts was
![]() and about
and about
![]() at quiescence.
at quiescence.
Tappert et al. (2013) reported a very interesting behavior of the
old Nova V728 Sco and presented some results of their analysis of
the star's spectral and photometric observations obtained in the
spring of 2012 at the La Silla observatory. It was 150 years
after the discovery of the Nova, and the star was very faint, at
the brightness level about
![]() . The observations were
acquired with the ESO NTT telescope in the Bessel
. The observations were
acquired with the ESO NTT telescope in the Bessel ![]() filter as a
part of a project to search for post-nova stars and form a sample
to compare their properties as a group. Based on the obtained
photometric data, light curves were constructed, indicating the
presence of a deep eclipse in the system. The orbital period was
determined to be
filter as a
part of a project to search for post-nova stars and form a sample
to compare their properties as a group. Based on the obtained
photometric data, light curves were constructed, indicating the
presence of a deep eclipse in the system. The orbital period was
determined to be
![]() . At the same time, V728 Sco showed
long-term variability, which the authors interpreted as small
outbursts like in dwarf novae. This fact and obtained
spectroscopic characteristics indicate a relatively low rate of
mass transfer in this system. Analysis of low-state eclipse data
provides strong, reliable evidence for the existence of a hot
inner disk, as predicted by Schreiber et al. (2000) for post-nova
stars. From the time of entry to the eclipse and exit from it, the
authors estimated the radius of the central object. This value
turned out to be larger than the radius of the white dwarf, and
the authors interpreted it as the radius of the hot inner region
of the accretion disk adjacent to the white dwarf.
. At the same time, V728 Sco showed
long-term variability, which the authors interpreted as small
outbursts like in dwarf novae. This fact and obtained
spectroscopic characteristics indicate a relatively low rate of
mass transfer in this system. Analysis of low-state eclipse data
provides strong, reliable evidence for the existence of a hot
inner disk, as predicted by Schreiber et al. (2000) for post-nova
stars. From the time of entry to the eclipse and exit from it, the
authors estimated the radius of the central object. This value
turned out to be larger than the radius of the white dwarf, and
the authors interpreted it as the radius of the hot inner region
of the accretion disk adjacent to the white dwarf.
Summarizing all the findings, Tappert et al. (2013) conclude that V728 Sco is a very interesting object characterized by unusual history. Therefore they suggested that this object could represent a special class of Novae.
We performed an analysis of photometric observations of V728 Sco obtained on 3 nights, kindly provided to us by Professor N. Vogt and his colleagues. The goal of our work was to determine main parameters of this variable using the method of the light curve solution developed by Khruzina (2011) and earlier used for analysis of photometric observations of different types of cataclysmic variables.
2. Description of the input parameters
The shape of the V728 Sco quiescent light curves is typical of dwarf novae. Such light curves are successfully reproduced within the framework of the standard "combined" model that takes into account the presence of a hot line near the lateral surface of the accretion disk and a hot spot on it on the leeward side of the jet (see Khruzina 2011).
Let us consider the main provisions of the "combined" model. The
system consists of a spherical star as a primary component
surrounded by an accretion disk, and a red dwarf as a secondary
completely filling its Roche lobe. The star is divided into 648
elementary surface areas radiating in accordance with its
temperature ![]() , depending on the effective temperature of the
secondary
, depending on the effective temperature of the
secondary ![]() . When calculating
. When calculating ![]() , the heating of the
surface of the secondary by radiation from the inner regions of
the accretion disk with a temperature of
, the heating of the
surface of the secondary by radiation from the inner regions of
the accretion disk with a temperature of ![]() ,
,
![]() is taken into account, where
is taken into account, where ![]() is the effective
temperature of the primary. The shape and size of the secondary
are set by the parameter
is the effective
temperature of the primary. The shape and size of the secondary
are set by the parameter ![]() . When calculating the
flow from elementary areas on the secondary's surface, the limb
darkening was taken into account in a linear approximation.
Gravitational darkening is described by the formula
. When calculating the
flow from elementary areas on the secondary's surface, the limb
darkening was taken into account in a linear approximation.
Gravitational darkening is described by the formula
![]() , where
, where ![]() is the local temperature,
is the local temperature,
![]() is the local acceleration of gravity on the surface of a
tidally deformed late-spectral-type star,
is the local acceleration of gravity on the surface of a
tidally deformed late-spectral-type star, ![]() (Lucy
1967). The brightness of an elementary area on the surface of the
star is described by the law
(Lucy
1967). The brightness of an elementary area on the surface of the
star is described by the law
![]() , where
, where ![]() is the angle between the normal to the elementary area on the
surface of the star and the line of sight;
is the angle between the normal to the elementary area on the
surface of the star and the line of sight;
![]() is the
coefficient of linear darkening to the edge;
is the
coefficient of linear darkening to the edge; ![]() is the Planck
function; and
is the Planck
function; and ![]() is the area of the considered elementary field.
The primary component is modeled by a sphere of radius
is the area of the considered elementary field.
The primary component is modeled by a sphere of radius ![]() ,
which is located in the focus of a weakly elliptical accretion
disk with an eccentricity
,
which is located in the focus of a weakly elliptical accretion
disk with an eccentricity ![]() , a large semi-axis
, a large semi-axis ![]() , and
orientation
, and
orientation ![]() (this is the angular distance in the
orbital plane between the periastron of the disk and the line
connecting the centers of mass of the components). The disk is
optically opaque and has a complex shape: it is geometrically thin
near the surface of the white dwarf, and geometrically thick at
the outer edge with an opening angle of
(this is the angular distance in the
orbital plane between the periastron of the disk and the line
connecting the centers of mass of the components). The disk is
optically opaque and has a complex shape: it is geometrically thin
near the surface of the white dwarf, and geometrically thick at
the outer edge with an opening angle of
![]() . The
temperature of each elementary area on the disk is determined by
the following relation:
. The
temperature of each elementary area on the disk is determined by
the following relation:
![]() , where
, where ![]() is the radius of the first
orbit near the primary,
is the radius of the first
orbit near the primary,
![]() , and the parameter
, and the parameter
![]() depends on the viscosity of the gas in the disk. In
the stationary state of the disk,
depends on the viscosity of the gas in the disk. In
the stationary state of the disk,
![]() ; with this
value decreasing, the flow from the disk increases significantly
due to a more flat distribution of the disk temperature along the
radius. When calculating the local temperature of the selected
elementary area on the disk, its heating by radiation from the
secondary component(this effect is usually insignificant) and
heating by high-temperature radiation from the internal areas of
the disk are taken into account. Details on other radiating
components (Khruzina 2011):
; with this
value decreasing, the flow from the disk increases significantly
due to a more flat distribution of the disk temperature along the
radius. When calculating the local temperature of the selected
elementary area on the disk, its heating by radiation from the
secondary component(this effect is usually insignificant) and
heating by high-temperature radiation from the internal areas of
the disk are taken into account. Details on other radiating
components (Khruzina 2011):
1. The area of collision of the gas flow with the disk, located
near its lateral surface. The interaction of the flow and the disk
is unstressed, the shock wave occurs in a narrow area along the
edge of the gaseous stream ("hot line"), as a result of
interaction of the incoming flows of the disk and the near-disk
halo with the jet material. The radiating region of the hot line
is represented with a truncated ellipsoid, the center of which is
located in the orbital plane inside the disk. The energy release
region in the applied model consists of two regions on the surface
of a truncated ellipsoid on its windward (
![]() ) and
leeward (
) and
leeward (
![]() ) sides outside the disk.
) sides outside the disk.
2. A hot spot on the side surface of the disk on the leeward side of the gaseous stream. Here, the radiating region is represented by a half-ellipse, whose center coincides with the point of intersection of the gas-flow axis with the disk. A detailed description of the special code is given in Khruzina (2011).
3. Results of modeling
To solve the inverse problem of determining parameters of the
system that has the shape of the synthesized light curve as close
as possible to the observed one, the Nelder-Meade method is used
(Himmelblau 1972). When searching for the global minimum of the
deviation, dozens of different initial approximations were set for
each of the light curves, since, the number of independent
variables in the studied parameter range being large, there
usually is a set of local minima. To assess the consistency of the
synthesized and observed light curves, the deviation is calculated
according to the formula within the framework of the applied
model:
where
Search for optimum model parameters for each light curve was
carried out for a grid of ![]() values in the range of
values in the range of ![]() ,
with increments
,
with increments
![]() ; orbital inclination
; orbital inclination ![]() in the
range of
in the
range of
![]() with increments
with increments
![]() ; and
; and
![]() in the range of
in the range of ![]() , with increments
, with increments
![]() , for a wide range of initial values
of other system parameters (
, for a wide range of initial values
of other system parameters (![]() is the distance between the
center of mass of the white dwarf and the inner Lagrangian point
is the distance between the
center of mass of the white dwarf and the inner Lagrangian point
![]() ,
, ![]() is the distance between the centers of mass of the
stars,
is the distance between the centers of mass of the
stars,
![]() is the half-thickness of the outer
edge of the disk in degrees).
is the half-thickness of the outer
edge of the disk in degrees).
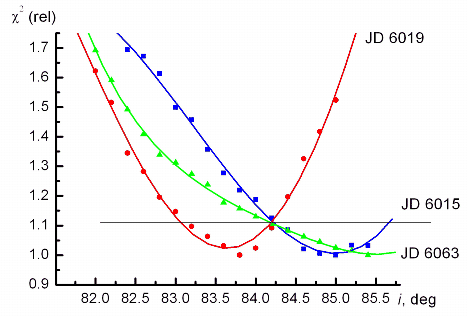
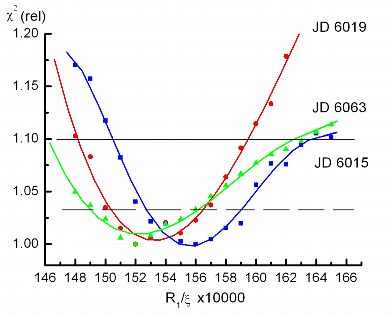
Using the obtained parameters, we constructed relations
![]() ,
, ![]() , and
, and
![]() (see figures
below). These figures show the ratio of the current deviation to
its minimum value,
(see figures
below). These figures show the ratio of the current deviation to
its minimum value,
![]() ,
for each of the observed light curves for a set of
,
for each of the observed light curves for a set of ![]() ,
, ![]() and
and
![]() .
.
| JD 2456015 ( |
JD 2456019 ( |
2456063 ( |
|||||||||
|
|
|
|
|
|
|
|
|||||
| 0.0055 | 19.1595 | .450E-02 | 19.17323 | 0.0033 | 20.7300 | .240E+00 | 21.04482 | 0.0069 | 19.38470 | .2335E-01 | 19.38379 |
| 0.0122 | 19.0850 | .301E-01 | 19.19493 | 0.0123 | 20.9250 | .130E+00 | 21.10337 | 0.0192 | 19.16700 | .6320E-01 | 19.21192 |
| 0.0166 | 19.0270 | .291E-01 | 19.20703 | 0.0237 | 21.0910 | .992E-01 | 20.98532 | 0.0261 | 19.04500 | .6520E-01 | 19.01641 |
| 0.0210 | 18.9530 | .261E-01 | 19.14194 | 0.0396 | 20.3367 | .111E+00 | 20.44740 | 0.0325 | 18.92400 | .8540E-01 | 18.78861 |
| 0.0256 | 18.8640 | .251E-01 | 19.00295 | 0.0487 | 19.7060 | .751E-01 | 19.40298 | 0.0386 | 18.66000 | .4330E-01 | 18.60223 |
| 0.0300 | 18.7530 | .231E-01 | 18.84699 | 0.0487 | 19.7060 | .751E-01 | 19.40298 | 0.0450 | 18.56800 | .3830E-01 | 18.34073 |
| 0.0344 | 18.6370 | .232E-01 | 18.66650 | 0.0532 | 19.5730 | .641E-01 | 19.33862 | 0.0512 | 18.48700 | .4940E-01 | 18.18702 |
| 0.0389 | 18.5050 | .192E-01 | 18.53165 | 0.0622 | 19.3397 | .127E-01 | 19.24793 | 0.0575 | 18.34100 | .4860E-01 | 18.08025 |
| 0.0433 | 18.4560 | .192E-01 | 18.41560 | 0.0759 | 19.2220 | .757E-02 | 19.21528 | 0.0637 | 18.18800 | .3860E-01 | 17.97565 |
| 0.0477 | 18.3450 | .182E-01 | 18.20781 | 0.0895 | 19.0800 | .362E-01 | 19.20376 | 0.0699 | 18.12300 | .3150E-01 | 17.90533 |
| 0.0522 | 18.2620 | .162E-01 | 18.13633 | 0.1009 | 18.8425 | .505E-01 | 18.99914 | 0.0762 | 18.02600 | .2740E-01 | 17.85583 |
| 0.0566 | 18.2110 | .152E-01 | 18.04809 | 0.1099 | 18.7055 | .450E-02 | 18.73841 | 0.0825 | 17.95900 | .2760E-01 | 17.80985 |
| 0.0610 | 18.1130 | .152E-01 | 17.96346 | 0.1235 | 18.6683 | .856E-02 | 18.69592 | 0.0887 | 17.85000 | .2450E-01 | 17.77662 |
| 0.0655 | 18.0550 | .142E-01 | 17.90536 | 0.1483 | 18.6516 | .888E-02 | 18.68874 | 0.0949 | 17.77000 | .2870E-01 | 17.75000 |
| 0.0699 | 17.9910 | .142E-01 | 17.85118 | 0.1824 | 18.6791 | .651E-02 | 18.65911 | 0.1011 | 17.68100 | .2470E-01 | 17.72908 |
| 0.0743 | 17.9210 | .132E-01 | 17.81820 | 0.2141 | 18.6540 | .743E-02 | 18.63882 | 0.1137 | 17.59230 | .1262E-01 | 17.70040 |
| 0.0797 | 17.8700 | .132E-01 | 17.78040 | 0.2391 | 18.6396 | .424E-02 | 18.61676 | 0.1354 | 17.57800 | .9860E-02 | 17.61980 |
| 0.0841 | 17.8250 | .132E-01 | 17.74932 | 0.4928 | 18.4558 | .133E-01 | 18.61292 | 0.7818 | 17.31880 | .7850E-02 | 17.32439 |
| 0.0908 | 17.7605 | .650E-02 | 17.72273 | 0.5157 | 18.5130 | .107E-01 | 18.61601 | 0.8210 | 17.25200 | .2031E-01 | 17.28038 |
| 0.0997 | 17.6980 | .340E-01 | 17.69294 | 0.6026 | 18.5004 | .749E-02 | 18.55894 | 0.8616 | 17.24670 | .1363E-01 | 17.25328 |
| 0.1085 | 17.6020 | .200E-01 | 17.67528 | 0.6350 | 18.5176 | .742E-02 | 18.52507 | 0.8968 | 17.26420 | .5220E-02 | 17.27126 |
| 0.1174 | 17.5420 | .300E-02 | 17.61019 | 0.6840 | 18.5487 | .159E-01 | 18.42737 | 0.9130 | 17.36200 | .3550E-01 | 17.31618 |
| 0.1418 | 17.5052 | .643E-02 | 17.53046 | 0.7205 | 18.4281 | .202E-01 | 18.33887 | 0.9225 | 17.44800 | .1700E-01 | 17.38146 |
| 0.1817 | 17.4816 | .697E-02 | 17.44590 | 0.7525 | 18.3084 | .263E-01 | 18.26824 | 0.9349 | 17.53750 | .1650E-01 | 17.49509 |
| 0.2183 | 17.4924 | .396E-02 | 17.43348 | 0.7843 | 18.2070 | .687E-02 | 18.21262 | 0.9442 | 17.67500 | .3190E-01 | 17.63548 |
| 0.2497 | 17.4594 | .126E-01 | 17.39734 | 0.8163 | 18.1659 | .130E-01 | 18.17520 | 0.9505 | 17.81000 | .4500E-01 | 17.72922 |
| 0.2985 | 17.4363 | .669E-02 | 17.40241 | 0.8516 | 18.1417 | .881E-02 | 18.15906 | 0.9568 | 17.96500 | .2750E-01 | 17.95679 |
| 0.3500 | 17.4295 | .107E-01 | 17.33711 | 0.8848 | 18.1443 | .767E-02 | 18.17093 | 0.9631 | 18.16000 | .2940E-01 | 18.11947 |
| 0.3836 | 17.3511 | .719E-02 | 17.31690 | 0.9121 | 18.1566 | .709E-02 | 18.20300 | 0.9693 | 18.26400 | .3540E-01 | 18.29915 |
| 0.4146 | 17.3319 | .437E-02 | 17.33547 | 0.9327 | 18.2090 | .808E-02 | 18.24765 | 0.9755 | 18.46000 | .3940E-01 | 18.42549 |
| 0.4457 | 17.2909 | .590E-02 | 17.33759 | 0.9465 | 18.3270 | .180E-01 | 18.32277 | 0.9819 | 18.64800 | .3420E-01 | 18.53766 |
| 0.4817 | 17.2867 | .214E-02 | 17.33966 | 0.9533 | 18.5850 | .302E-01 | 18.39284 | 0.9882 | 18.84700 | .5730E-01 | 18.89410 |
| 0.5210 | 17.2832 | .464E-02 | 17.33874 | 0.9578 | 18.8610 | .312E-01 | 18.73218 | 0.9944 | 19.11600 | .7120E-01 | 19.06111 |
| 0.5483 | 17.2855 | .866E-02 | 17.33869 | 0.9624 | 19.0190 | .341E-01 | 18.85286 | ||||
| 0.5806 | 17.2912 | .318E-02 | 17.33273 | 0.9714 | 19.4030 | .379E-01 | 19.21883 | ||||
| 0.6200 | 17.2971 | .695E-02 | 17.31517 | 0.9806 | 19.5910 | .731E-01 | 19.54504 | ||||
| 0.6496 | 17.3474 | .654E-02 | 17.32491 | 0.9852 | 19.8970 | .103E+00 | 19.67583 | ||||
| 0.6807 | 17.3752 | .505E-02 | 17.32615 | 0.9965 | 20.5160 | .160E-01 | 20.53229 | ||||
| 0.7155 | 17.3380 | .865E-02 | 17.27641 | ||||||||
| 0.7457 | 17.3046 | .640E-02 | 17.26143 | ||||||||
| 0.7931 | 17.2501 | .622E-02 | 17.23598 | ||||||||
| 0.8371 | 17.1963 | .643E-02 | 17.21017 | ||||||||
| 0.8615 | 17.1730 | .763E-02 | 17.20130 | ||||||||
| 0.8814 | 17.1990 | .801E-02 | 17.20518 | ||||||||
| 0.8970 | 17.2402 | .851E-02 | 17.22025 | ||||||||
| 0.9132 | 17.3452 | .156E-01 | 17.26524 | ||||||||
| 0.9287 | 17.4607 | .561E-02 | 17.34989 | ||||||||
| 0.9455 | 17.7230 | .133E-01 | 17.54469 | ||||||||
| 0.9500 | 17.7930 | .133E-01 | 17.59658 | ||||||||
| 0.9544 | 17.8780 | .143E-01 | 17.65476 | ||||||||
| 0.9589 | 17.9810 | .143E-01 | 17.81286 | ||||||||
| 0.9633 | 18.0820 | .152E-01 | 17.94045 | ||||||||
| 0.9677 | 18.2010 | .172E-01 | 18.03418 | ||||||||
| 0.9722 | 18.2850 | .172E-01 | 18.15612 | ||||||||
| 0.9788 | 18.4180 | .160E-01 | 18.32101 | ||||||||
| 0.9855 | 18.5560 | .202E-01 | 18.64906 | ||||||||
| 0.9900 | 18.7150 | .232E-01 | 18.71060 | ||||||||
| 0.9944 | 18.8100 | .251E-01 | 18.76013 | ||||||||
| 0.9989 | 19.0450 | .291E-01 | 19.05699 | ||||||||
Figures 1-3 show the ratio of the relative deviation to its
minimum value for each of the curves, for different mass ratios
![]() , orbital inclinations
, orbital inclinations ![]() , and radii of the primary component.
, and radii of the primary component.
Considering the relations we obtained, it is possible to evaluate
the basic system parameters:
Effective temperatures we use (averaged over the surface of stars) can change at different times. For example, if there are dark spots on the surface of the secondary, the average temperature will be lower than in the case of their absence. The same situation is with a white dwarf in the case of the presence of a bright accretion zone on its surface. Thus, temperatures of the stars were not fixed and were the desired parameters.
We also searched for main parameters of the other system components (temperatures and sizes of the disk, hot line, and hot spot); they are collected in Table 2.
| Parameters | JD 2456015 | JD 2456019 | JD 2456063 |
| 2.5 | 2.5 | 2.5 | |
|
|
84.2 | 84.2 | 84.2 |
| 0.593(3) | 0.593(3) | 0.593(3) | |
| 0.312(3) | 0.312(3) | 0.312(3) | |
| 0.0154(2) | 0.0154(2) | 0.0154(2) | |
| 0.0091(1) | 0.0091(1) | 0.0091(1) | |
| Disk | |||
| 0.003(1) | 0.023(2) | 0.016(5) | |
|
|
113.35(2) | 112.2(3) | 112.6(6) |
|
|
0.843(4) | 0.598(3) | 0.879(2) |
| 0.498(2) | 0.347(3) | 0.513(1) | |
|
|
0.60(4) | 0.51(1) | 0.31(1) |
| 0.557(1) | 0.664(5) | 0.477(2) | |
| Hot spot | |||
|
|
0.44(2) | 0.29(2) | 0.37(2) |
| Hot line | |||
| 0.093(1) | 0.046(1) | 0.048(1) | |
| 0.297(1) | 0.407(1) | 0.289(3) | |
| 0.003(1) | 0.003(1) | 0.003(1) | |
|
|
27.0(1) | 48.1(3) | |
|
|
|||
|
|
|||
| 4579 ( |
799 ( |
404 ( |
The observed light curves and those synthesized with the parameters from Table 2 are displayed in Fig. 4.
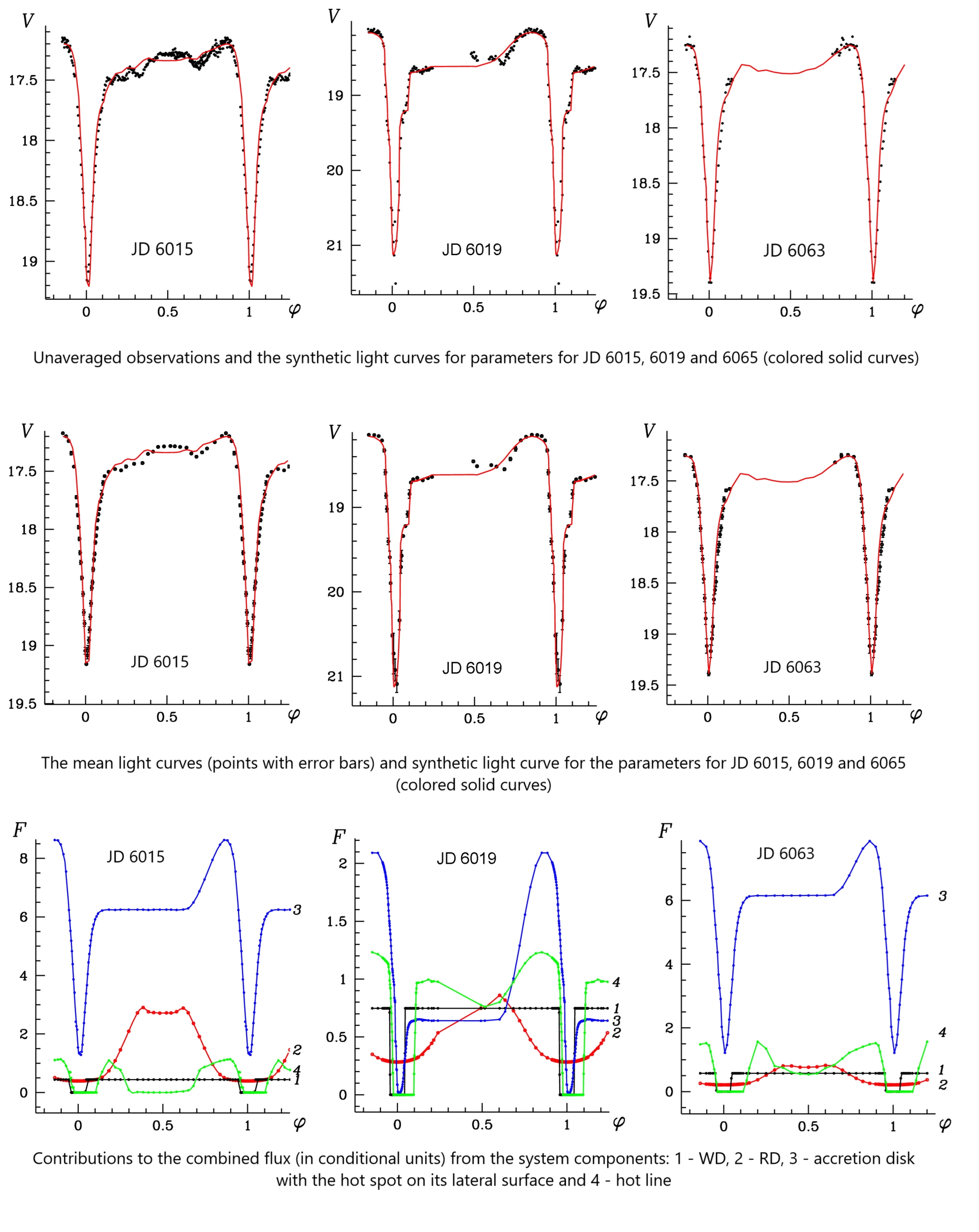 |
Fig. 4. Folded light curves of V728 Sco and analysis of the contribution of components to them. Truncated Julian dates (with leading figures 245... omitted) are indicated. |
Figure 5 presents a schematic view of the components of V728 Sco,
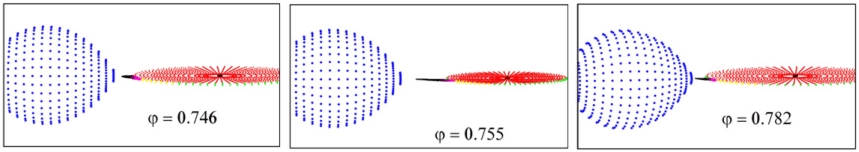 |
Fig. 5. Schematic views of V728 Sco. Blue: the red dwarf; black: the white dwarf; red: inner disk; green: lateral side of the disk; yellow: hot spot; black: cold part of the hot line; magenta with gradient: heated part of the hot line. |
Determining the parameters of the V728 Sco system using the Khruzina code not only confirms its usefulness in the case of analyzing photometric data for cataclysmic variables, making this determination more accurate and reliable; the code makes it possible to use the entire light curve for analysis, and not only the eclipse area (as in the case of spectroscopic observations), i.e. it is based on more numerous data. The method was already successfully applied to the analysis of light curves of many dwarf novae and showed good results.
4. Summary
1. We analyzed three observational curves light curves of the cataclysmic variable V728 Sco, believed to be an old Nova, obtained during different stages of its activity1.
2. We derived the system parameters of V728 Sco. The shape of the synthetic light curve matches the observed one most closely.
3. Contributions to the combined system flux from the different components, such as white dwarf, red dwarf, accretion disk with the hot spot on its lateral surface, and hot line were determined in the frame of combined model (Khruzina 2011) for active and quiet states.
4. The undoubted advantage of the Khruzina code of light curve solution is the use of the entire light curve for determination of system parameters, and not just the area of the eclipse.
Acknowledgements. The study was conducted under the state
assignment of Lomonosov Moscow State University.
The authors are very grateful to Dr. C. Tappert, Dr. L. Schmidtobreick, and Prof. N. Vogt for providing photometric observations of V728 Sco.
A. Tarasenkov acknowledges the support of the Foundation for the Development of Theoretical Physics and Mathematics BASIS (project 24-2-1-6-1).
References:
Himmelblau, D. 1972, Applied nonlinear programming, Russian translation: 1975, Moscow: Mir Publishers, p. 163
Khruzina, T. S. 1998, Astron. Rep., 49, No. 10, 783
Khruzina, T. S. 2011, Astron. Rep., 55, No. 5, 425
Lucy, L. B. 1967, Zeitschrift für Astrophysik, 65, H. 2, 89
Schreiber, M. R., Gänsicke, B. T., & Cannizzo, J. K. 2000, Astron. & Astrophys., 362, No. 1, 268
Tappert, C., Ederoclite, A., Mennickent, R. E., et al. 2012, Monthly Notices Roy. Astron. Soc., 423, No. 3, 2476
Tappert, C., Vogt, N., Schmidtobreick, L., et al. 2013, Monthly Notices Roy. Astron. Soc., 431, No. 1, 92
Tebbutt, J. 1878, Monthly Notices Roy. Astron. Soc., 38, No. 5, 330